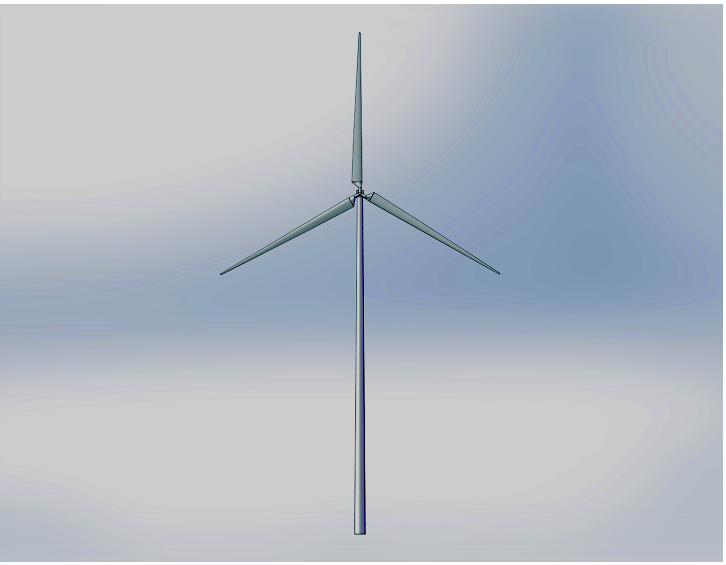
图1 风力发电机模型
Fig.1 Wind turbine model
摘要 风能是一种快速发展的清洁能源,但是雷电威胁风电场的安全稳定运行。区别于以往简化物理过程的模型,该文使用上行先导起始及发展模型(SLIM)对上行先导的起始以及发展全过程进行计算分析,得出了3 MW风机闪击距离和雷电屏蔽距离的定量结果。同时区别于静止结构,该文还分析了不同旋转角度对风机闪击距离的影响。分析得出,在考虑上行先导的情形下,闪击距离不仅与回击电流相关,也会随着叶片旋转角度的减小和下行先导偏移量的减小而减小。根据定义的相邻风机雷电屏蔽距离,基于闪击距离的计算结果分析不同旋转角度、大气湿度、大气压强下的雷电屏蔽效应。结果表明,旋转角度的增大会导致雷电屏蔽距离减小;同时大气湿度和压强的增大,都会增大雷电屏蔽距离,基于此可为风机防雷布置提供参考。
关键词:风力发电机群 上行先导 雷电防护 屏蔽效应 环境参数分析
为了响应“碳达峰·碳中和”目标,新能源发电在世界各地得到了大力发展[1-2]。风能作为最重要的一种新能源,在我国得到了迅猛发展,截至2023年,我国风电装机总量超过4.4亿kW,占全球累计风电装机总量的42.2%。为了适应需求,风电场规模越发扩大,风机容量随之提升,风机叶片的尺寸也不断增加[3],且部分风电场位于高原地区和海上,也给风电场的维护带来了困难[4]。因此,雷电正在且将越发显著地影响风电场的安全稳定运行。
雷电是一种具有强大破坏性的放电现象,严重威胁电力系统的安全可靠运行[5-6],其具有大电流、高电压的特点,会给输电线路[7-8]和风力发电机[9]造成不同程度的损坏,以及严重的经济损失。关于风力发电机雷击特性和雷电防护的研究主要是基于自然观测[10]、仿真实验[11-13]、实验室缩比试验[3]、单叶片试验[14]和材料放电试验[15]。基于人工触发闪电的试验方法也在最近得到开展,但难以大量进行,仍需进一步研究[16]。
由于风机高大且快速旋转的特性,风机遭受雷击的风险与上行闪电联系更加紧密[17]。经过观测发现,上行闪电由于其所涉及的电流普遍较低,可能未被雷电检测装置监测,因此目前与风机相关的上行闪电次数可能被严重低估[18]。相对来说,上行闪电并不常见,只起始于山顶或高大的建筑物上[19],因此对上行闪电的研究起步相对较晚[20]。对上行闪电的观测表明,上行闪电可以分为两类[21]:一类是自触发类型,在此类上行闪电周围无其他放电活动;另一类是其他触发类型,此类上行闪电是受到附近闪电活动的触发而起始的,如响应下行先导的临近。上行先导的速度通常低于下行先导,一般为104~105 m/s[22]。风力发电机一般以机群的形式出现,多个上行先导同时发生的事件也有相关报道[23],因此由感应而产生的上行闪电的概率会远远大于遭受直击雷的概率[24]。
在雷电防护领域,以往采用的电气几何模型忽略了上行先导的发展,大大简化了雷电附着的物理过程,低估了闪击距离随着上行先导发展的变化,从而会低估雷电对建筑物或者电气设备的危害。近年来,考虑上行先导的雷电防护模型在输电线路[25-26]和风力发电机[27-28]上得到广泛运用,并通过与实际结果对照验证了模型的准确性,充分说明了考虑上行先导的发展是评估雷电过程的重要组成部分。黄胜鑫等[29]通过上行先导起始模型对上行先导的雷电截收面积进行了计算。在此基础上,Nie Jiayi等[30]考虑了地形对雷电截收面积的影响。但是上行先导起始模型并未完整地模拟整个接闪过程,忽略了上行先导的后续发展,其结果的准确性取决于接闪判据的准确性。Zhang Li等[31]采用上行先导起始及发展模型(the Self-consistent Leader Inception and propagation Model, SLIM)对电气几何模型进行修正,但是并未考虑下行先导发展带来的背景电势变化。Yang Ning等[32]通过SLIM分析了上行先导发展对吸引半径的影响,但是并未考虑不同叶片旋转角度带来的差异,无法反映风机相对于静止高塔的差别。考虑上下行先导发展的完整物理过程将在本文得到研究。
根据上述观测结果与理论计算分析,本文将基于上行先导起始及发展模型对风力发电机的雷电闪击距离进行计算,并分析临近情况下的上行雷电屏蔽效应,为风电场的安全稳定运行提供参考。
参考相关数据[17],建立3 MW风力发电机模型如图1所示,其中叶片长度为45 m,塔筒长度为95 m。根据Zhou Qibin等[12]的研究,叶尖接闪器的尺寸定为50 mm2。

图1 风力发电机模型
Fig.1 Wind turbine model
为了模仿最易于上行先导发展的雷云条件,本文采用两个带电圆柱形区域模拟雷暴云结构。正电荷区底部距离地面8 km,区域垂直厚度为3 km,水平半径为4 km,电荷密度为3 nC/m3;负电荷区底部距离地面4 km,区域垂直厚度为4 km,水平半径为4 km,(负)电荷密度为-1 nC/m3。仿真区域设置为边长为4 000 m的正方体区域,地面采用狄利克雷边界条件,满足电势φ=0;周围边界采用诺伊曼边界条件,满足∂φ/∂n=0。
下行先导采用线电荷模型,其线电荷密度如式(1)所示[33],已通过与人工触发闪电的试验参数对比证明了公式的准确性。
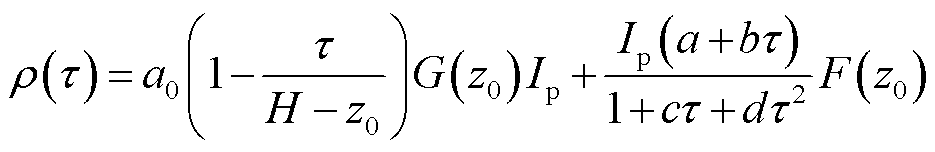 (1)
(1)
其中
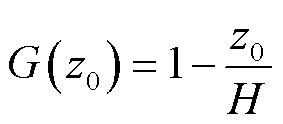 (2)
(2)
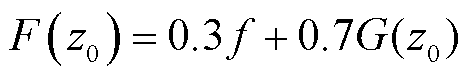 (3)
(3)
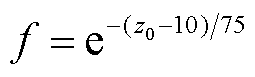 (4)
(4)
式中,ρ(τ)为下行先导通道线电荷密度,C/m;τ为下行先导通道中某点到先导头部的距离,m;z0为下行先导头部距离地面的高度,m;H为雷云高度,取H=4 000 m;Ip为回击电流峰值,kA;a0、a、b、c、d均为系数,分别为a0=1.476×10-5、a=4.857×10-5、b=3.909 7×10-6、c=0.522、d=3.73×10-3。下行先导电荷分布随先导头部高度的变化趋势如图2所示。可以看出,先导电荷主要集中于头部,且随着先导的发展,头部电荷会逐渐增大。下行先导的发展速度设定为恒定值4×105 m/s。
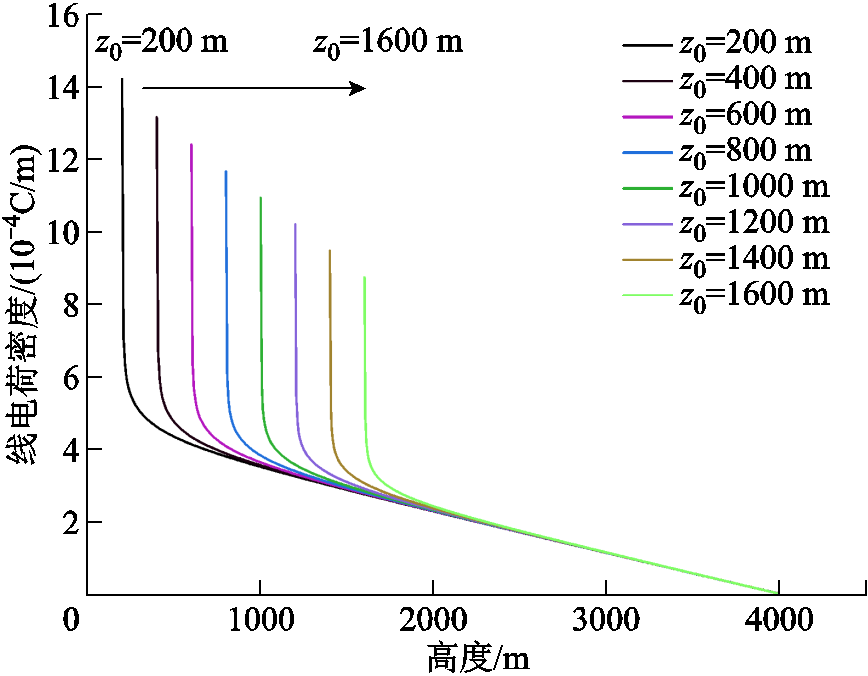
图2 下行先导电荷分布随先导头部高度变化趋势
Fig.2 Trend of downward leader charge distribution with the variation in leader tip height
由于90%的自然闪电都是负极性的,因此本文针对负极性的下行先导进行分析。正极性上行先导起始模型主要基于M. Becerra和V. Cooray提出的简化物理模型[34],其准确性在人工触发闪电[34]、自然观测[32]和雷电定位系统[30]中均得到了验证。电晕的起始判据是达到起晕场强,并且当流注区的电晕电荷累计到1 μC时则认为满足电晕爆发条件,电晕爆发后视为不稳定的先导起始。电晕形成后,将流注区按照线性电势分布进行建模。电晕区域的空间电荷可通过引入几何因子进行简化,以提高计算效率,表示为
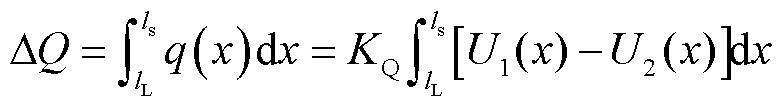 (5)
(5)
式中,ls为电晕区的长度;lL为正极性上行先导的长度;q为电晕区的电荷分布;U1和U2分别为电晕形成前后电晕区的电势分布;KQ为几何因子。第i步上行先导尖端的电势可计算为
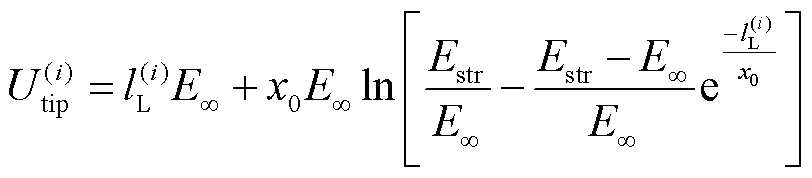 (6)
(6)
式中,E∞为稳定状态下正极性先导通道电场强度;x0为常数,由正极性先导速度v和时间常量θ相乘得到;Estr为正极性流注通道电场强度。在分析先导起始过程中,因为其时间很短,可以假设空间电势不发生变化。而先导的发展量由每一步迭代产生的电荷量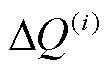 除以单位长度所需的电荷量qL得到,即
除以单位长度所需的电荷量qL得到,即
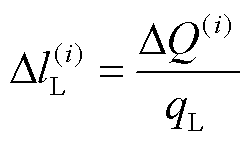 (7)
(7)
当上行先导增长到2 m时停止迭代,认为上行先导稳定起始,开始分析上行先导的发展;若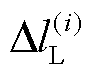 <0,认为上行先导停止发展,下行先导步进发展。
<0,认为上行先导停止发展,下行先导步进发展。
由于上行先导的起始时间极短,仅为数十微秒[35],所以在分析上行先导起始阶段时可以考虑下行先导静止。但是上行先导的发展需要考虑下行先导的步进,当下行先导接近时,背景电势分布将发生变化。这种受电晕放电和空间电势变化双重影响的效应反映在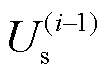 对
对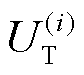 的扭曲上,用公式表示为
的扭曲上,用公式表示为
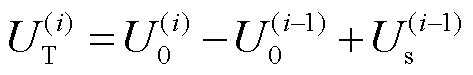 (8)
(8)
式中,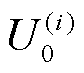 为环境产生的背景电势分布;
为环境产生的背景电势分布;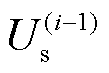 为第i-1次计算后电晕产生的空间电势分布;
为第i-1次计算后电晕产生的空间电势分布;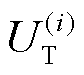 为第i个计算步骤时环境产生的背景电势和第i-1个计算步骤时电晕产生的空间电势共同产生的空间电势分布。空间电势变化下的空间电荷计算示意图如图3所示。通过分析可以近似得到每一步上行先导尖端产生的电荷量为
为第i个计算步骤时环境产生的背景电势和第i-1个计算步骤时电晕产生的空间电势共同产生的空间电势分布。空间电势变化下的空间电荷计算示意图如图3所示。通过分析可以近似得到每一步上行先导尖端产生的电荷量为
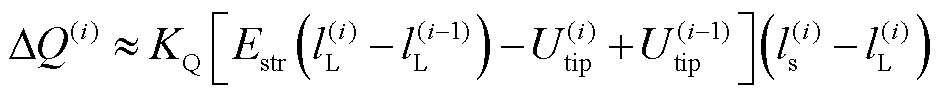 (9)
(9)
考虑上行先导发展模型的风机叶片接闪距离计算流程如图4所示。下行先导步进会使得背景电势发生变化,从而电晕放电会更加激烈。迭代计算所需参数及其定义见表1,参数的取值源自长气隙放电试验[36-37]。由于对于正极性先导放电的研究较为初步,相关自然观测的结果难以获取,而实验室放电试验能够反映电流不是很大的上行先导放电,同时相关参数的测量也更加精确。
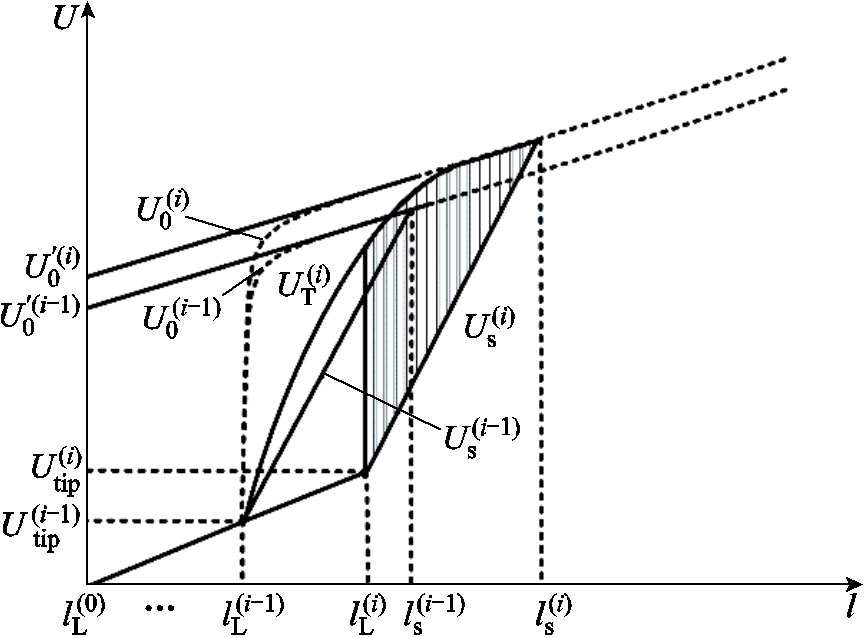
图3 空间电势变化下的空间电荷计算示意图
Fig.3 Schematic representation of space charge calculation under spatial potential variation
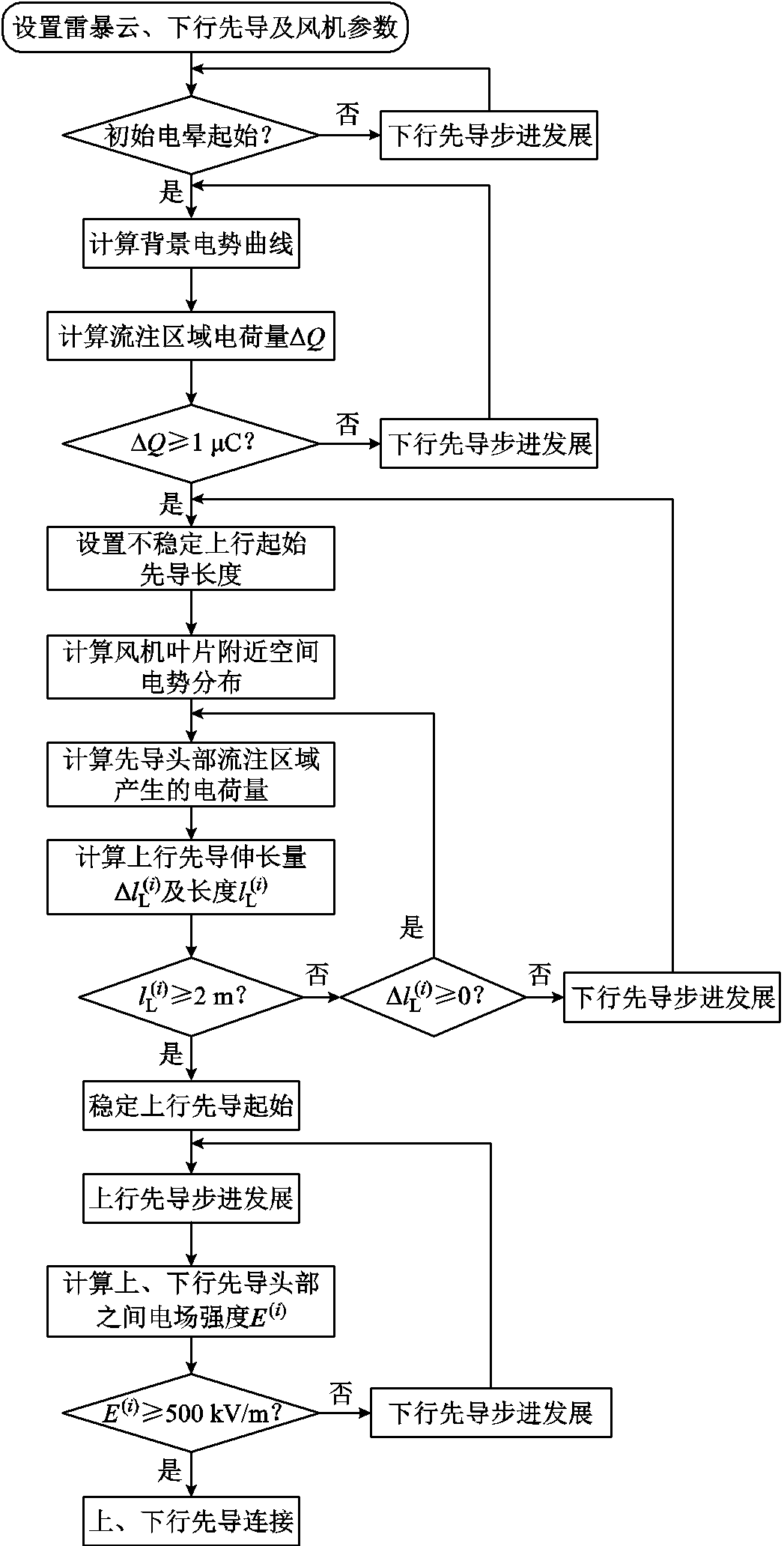
图4 考虑上行先导发展模型的风机叶片闪击距离计算流程
Fig.4 Flow chart for calculating striking distance of wind turbine blades considering upward leader development model
表1 迭代运算所需参数
Tab.1 Parameters required for iterative calculation

参数数值 初始先导长度/m5×10-2 正极性流注通道电场强度Estr/(V/m)4.5×105 正极性先导通道电场强度3×104 正极性先导特性常数0.75 先导通道线电荷密度65×10-6 先导通道几何因子3.5×10-11
本文定义当上、下行先导满足击穿条件,即两者之间的平均空间电场强度达到500 kV/m时[38],下行先导距风机叶片尖端的距离为闪击距离,如图5所示。由于风机叶片是计算模型中的唯一高点,且叶片尖端是最常见的雷击附着点[39],因此本文的雷击点仅设为叶片尖端。同时当下行先导尖端与地面之间的平均电场强度达到500 kV/m时,下行先导与地面发生击穿。
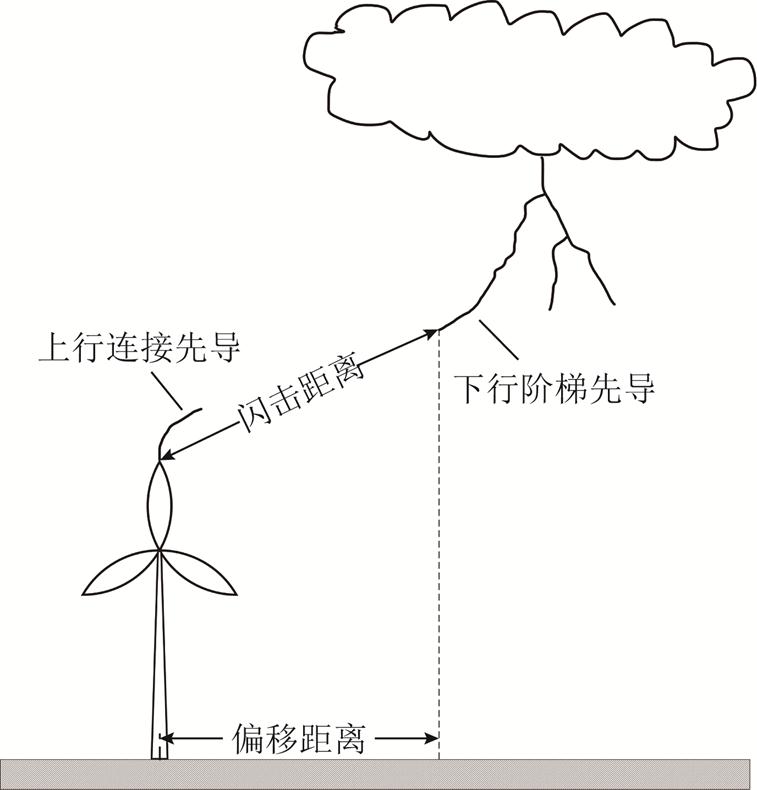
图5 风力发电机闪击距离定义
Fig.5 Definition of striking distance for wind turbine
定义风机叶片竖直向上时旋转角度为90°,分别计算风机叶片旋转90°、60°和30°时上、下行先导连接点的高度及闪击距离,如图6~图8所示。需要注意的是,根据M. Becerra的研究[40],电晕电荷的存在仅对电晕放电过程存在影响,对后续的先导发展基本不存在影响。而本文的主要内容是先导的发展,虽然电晕和流注放电确实受到电荷分布的影响,但是其影响的是先导稳定起始的时刻,这一过程相对于后续先导发展的时间很短,因此本文不讨论电晕电荷随流场变化的影响。通过计算结果可以发现:随着下行先导偏移距离的增大,雷击时连接点的高度会逐渐减小,且回击电流峰值越大,连接点越高,这也能反映接闪器的保护范围随着回击电流峰值的增大而增大,风机遭受雷击的概率也会大大提升。而实际情况中,回击电流峰值越大,说明下行先导通道中的电荷越多,故而叶片附近的空间电场得到增强,接闪器上也更容易产生稳定的上行先导,上、下行先导头部之间的电场强度也更大,最终导致雷击时上、下行先导连接点会出现在更高处。
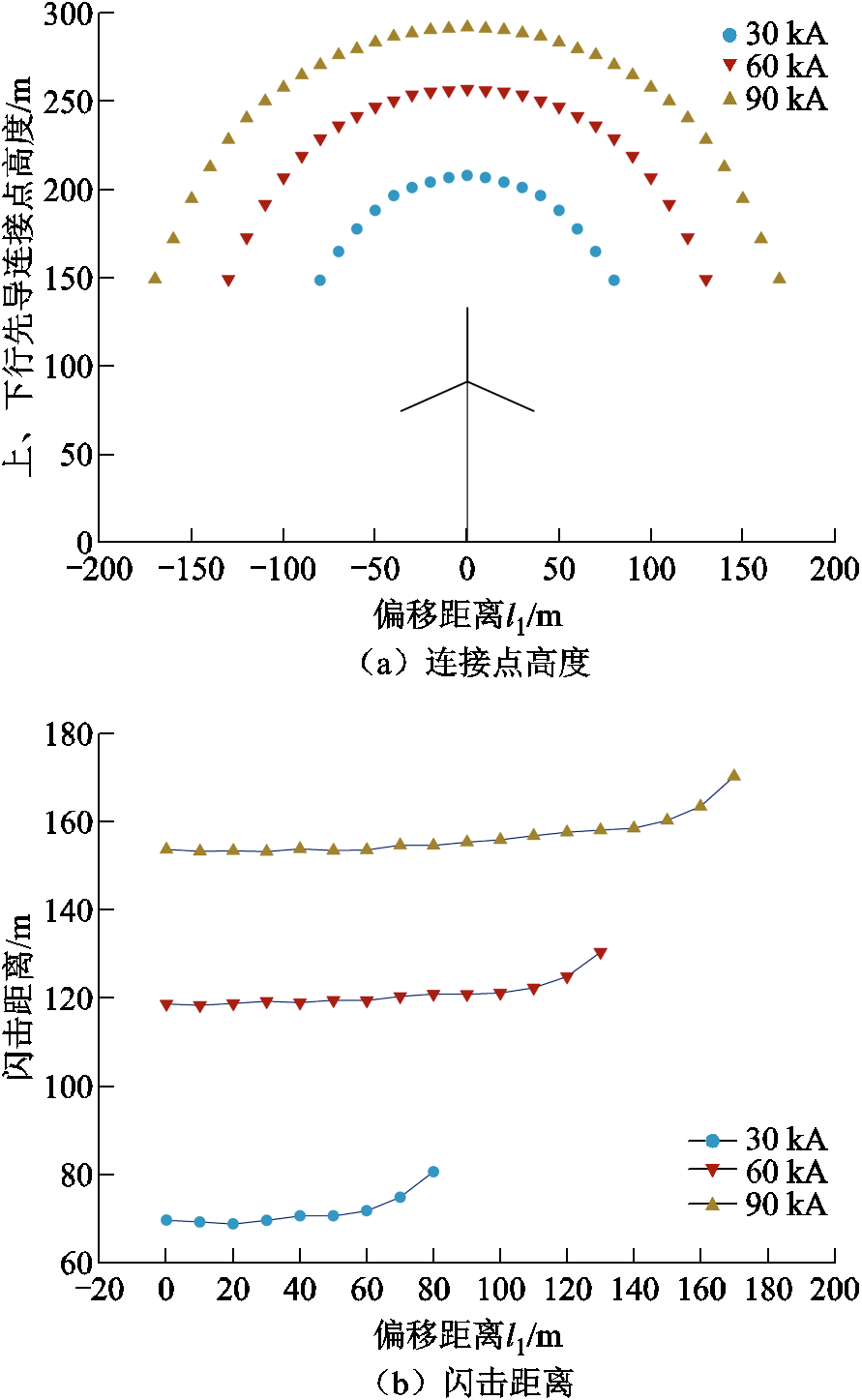
图6 不同回击电流下叶片旋转90°时上、下行先导连接点高度及闪击距离
Fig.6 Coordinates of upward and downward leader connection points and striking distances at a rotation angle of 90° under different return stroke currents
闪击距离并不是恒定值,其会随着回击电流峰值和风机旋转角度的变化而变化。选取旋转角度为90°的情况分析电流峰值变化对闪击距离的影响。当偏移距离为0时,回击电流峰值30 kA下的闪击距离为69.7 m,60 kA下的闪击距离为118.7 m,90 kA下的闪击距离为153.8 m。随着电流幅值的增大,闪击距离逐渐增大,但增长率呈现减小的趋势。这是因为随着回击电流的增大,下行先导尖端电势变大,会导致上、下行先导之间更早发生击穿。风机叶片旋转角度增大,闪击距离也会随之增大。这是因为旋转角度越大,接闪器越远离地面,在同样的下行先导条件下,风机叶尖的电场强度就会越大,故上行先导会得到更好的发展。当偏移距离为0时,选取回击电流峰值为30 kA,当旋转角度由90°变为60°时,闪击距离由69.7 m变为67.78 m,变化不大;而当旋转角度由60°变为30°时,闪击距离变为55.1 m。这是因为接闪器越靠近地面,尖端电势变化越大。
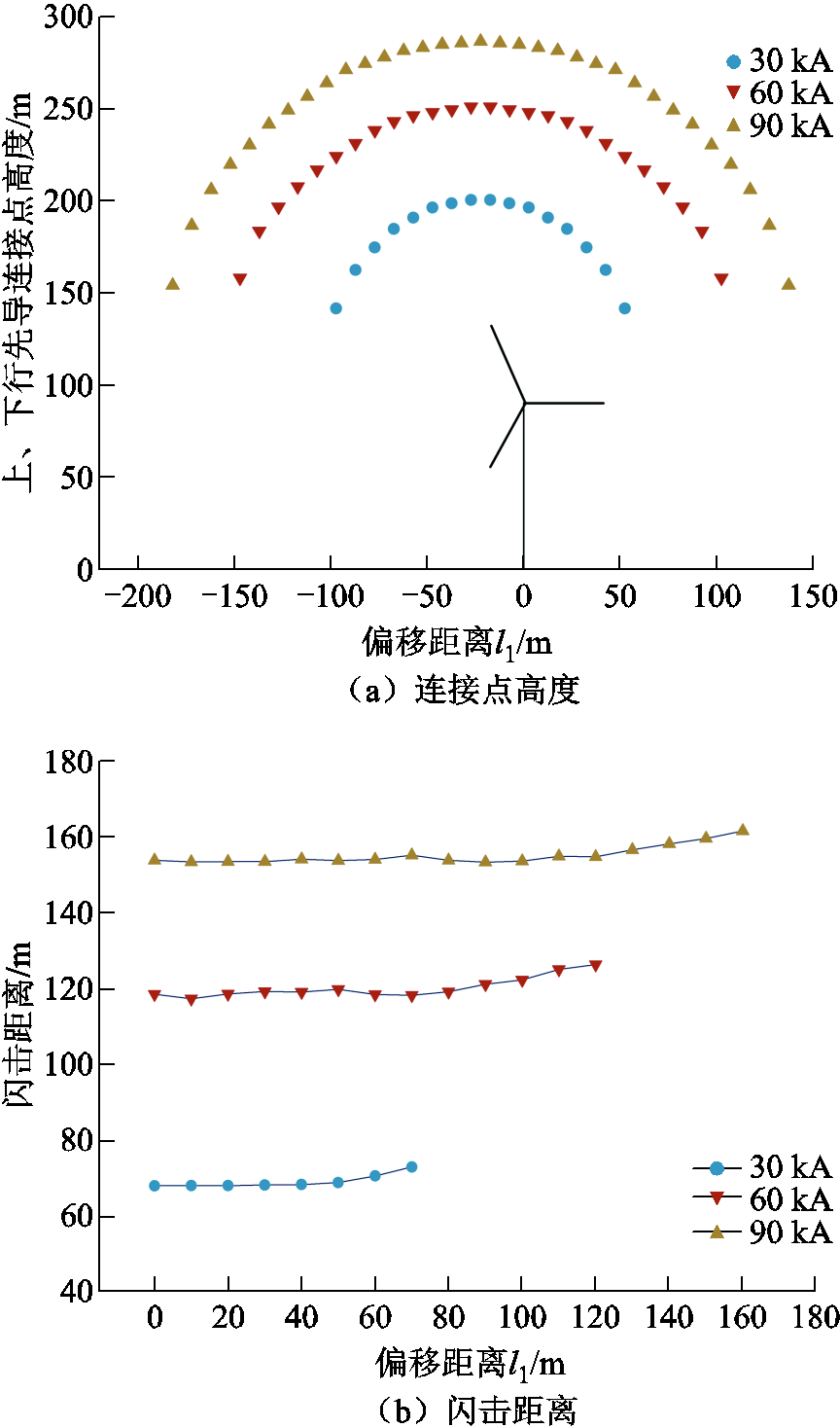
图7 不同回击电流下叶片旋转60°时上、下行先导连接点高度及闪击距离
Fig.7 Coordinates of upward and downward leader connection points and striking distances at a rotation angle of 60° under different return stroke currents
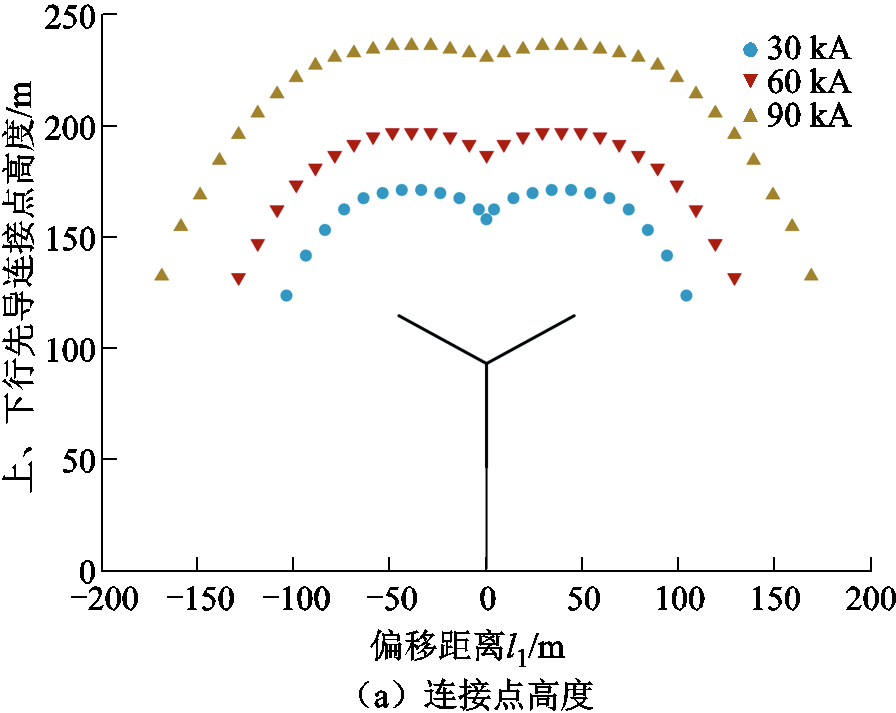
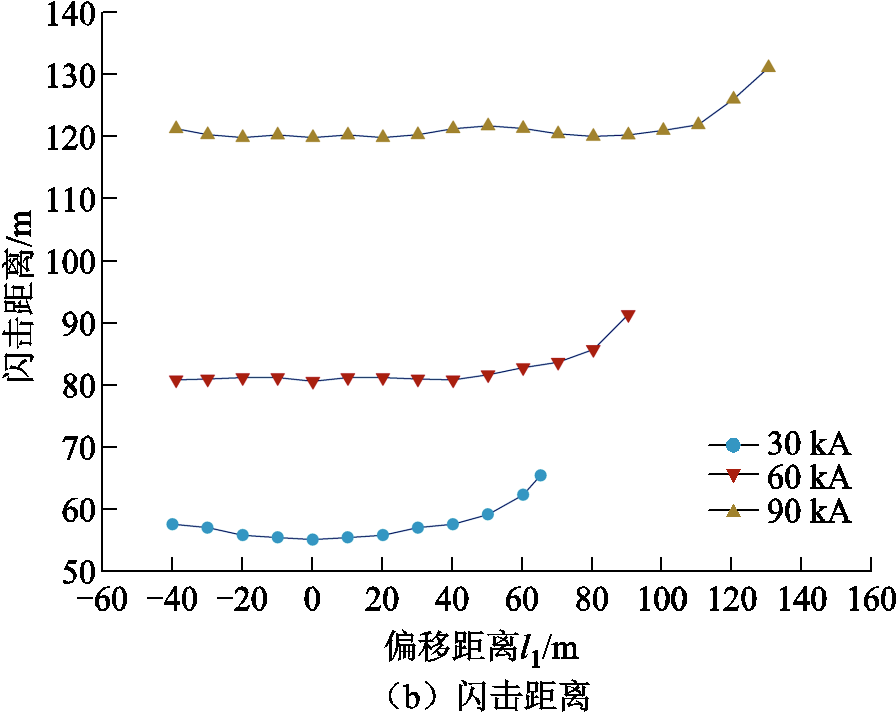
图8 不同回击电流下叶片旋转30°时上、下行先导连接点高度及闪击距离
Fig.8 Coordinates of upward and downward leader connection points and striking distances at a rotation angle of 30° under different return stroke currents
闪击距离随着下行先导的偏移也会发生改变。当偏移距离较小时,闪击距离变化不大;而当偏移距离较大时,闪击距离会变大。这是因为随着下行先导的偏移,给予上行先导更长的发展时间,上行先导不会过早地与下行先导之间发生击穿,能够在更远的距离下进行接闪。选取回击电流峰值为 30 kA且旋转角度为90°的情况,当偏移距离较小时,闪击距离变化不大,而随着偏移距离逐渐增大,闪击距离开始逐渐增加且增长率逐渐增大。由图6看出,下行先导未偏移时的闪击距离为69.7 m,而当偏移距离逐渐增大至70 m后,在计算上、下行先导连接点与接闪器的几何距离时,偏移距离会显著地增大闪击距离。
总而言之,闪击距离的变化取决于上行先导的发展,当不考虑上行先导时,闪击距离就取决于回击电流峰值的恒定值;当考虑上行先导发展时,上行先导的发展越明显,闪击距离将越大。
根据2.1节中的分析,下行先导的偏移也会产生上行先导。因此当下行先导临近风电场时,多个风机都会发生上行先导起始。以双风机的布置为例对风力发电机的雷电屏蔽效应进行分析,水平方向屏蔽距离和最大屏蔽距离示意图如图9所示。假设两个风机叶片关于中心轴线呈轴对称分布。浅蓝色的圆形区域代表风机叶片在当前旋转角度下对雷电的吸引范围,圆心位于叶片尖端的接闪器上,半径为风机叶片的闪击距离Rs。图9中,R为风机叶片的长度,θ为风机叶片和水平方向的夹角,D为两个风机之间的间距。由几何关系可以得出风机叶片在水平方向的屏蔽距离Lx和风机最大屏蔽距离Dmax的计算公式[31]为
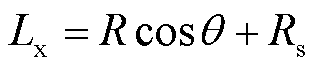 (10)
(10)
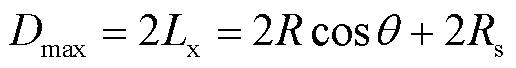 (11)
(11)
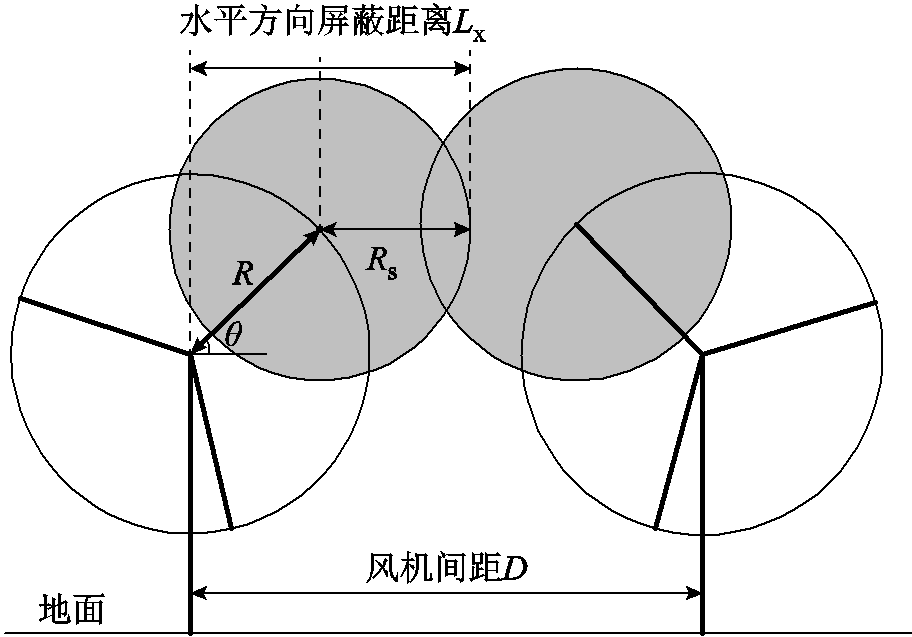
图9 水平方向屏蔽距离和最大屏蔽距离示意图
Fig.9 Schematic diagram of horizontal shielding distance and maximum shielding distance
不同回击电流峰值、旋转角度下的最大屏蔽距离计算结果如图10所示。在同样的叶片旋转角度下,雷电流幅值越大,最大屏蔽距离也越大。但是在回击电流峰值为30 kA时,叶片旋转角度为30°所对应的最大屏蔽距离最大,其次是60°,90°时的最大屏蔽距离最小;在回击电流峰值为60 kA和90 kA时,叶片旋转角度为60°所对应的最大屏蔽距离最大,其次是30°,90°时的最大屏蔽距离最小。这意味着当风力发电机叶片旋转时,对临近风机的保护效果会减弱,这个结果取决于闪击距离在水平方向上的投影。当不考虑上行先导时,雷电屏蔽效应会明显减弱。
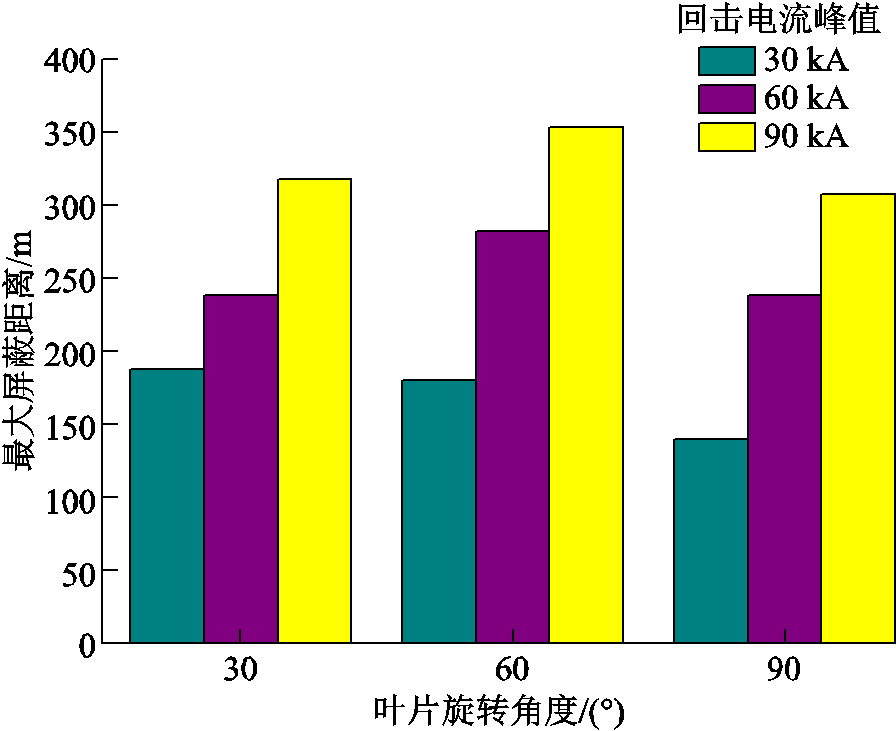
图10 不同回击电流峰值、旋转角度下的最大屏蔽距离
Fig.10 Maximum shielding distance under different return stroke current and rotation angles
陆上风电场常常位于高原地带,海上风电场的规模也越发扩大,因此,研究环境参数对风电场风机雷电屏蔽效应的影响也越发关键。
正极性流注的电场强度Estr是计算的关键参数,其会受到湿度以及相对空气密度的共同影响[41],即
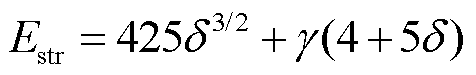 (12)
(12)
式中,γ为绝对空气湿度,g/m3;δ为相对空气密度,表达式为
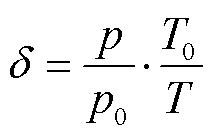 (13)
(13)
式中,p为空气压强,kPa;T为温度,K;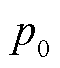 和T0分别为标准大气条件下海平面的气压和温度,
和T0分别为标准大气条件下海平面的气压和温度,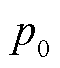 = 1 atm=101 kPa,T0=293 K。最大屏蔽距离Dmax随气压、温度、空气湿度的变化如图11所示。可以看出,随着气压、湿度的增大和温度的降低,雷电屏蔽效应会逐渐增大。
= 1 atm=101 kPa,T0=293 K。最大屏蔽距离Dmax随气压、温度、空气湿度的变化如图11所示。可以看出,随着气压、湿度的增大和温度的降低,雷电屏蔽效应会逐渐增大。
气压、温度、空气湿度三者与海拔 的关系为[42]
的关系为[42]
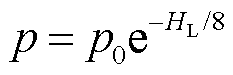 (14)
(14)
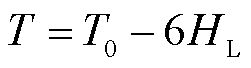 (15)
(15)
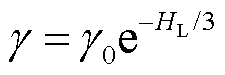 (16)
(16)
式中,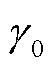 为标准大气条件下海平面的绝对湿度,
为标准大气条件下海平面的绝对湿度,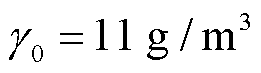 。
。
雷电屏蔽距离随着海拔的变化趋势如图12所示,当海拔从0上升到2 km范围内时,Dmax几乎呈现线性衰减;随着海拔继续上升,Dmax减小的趋势会更加明显,尤其是在海拔从3 km上升到4 km时,Dmax减小了33.2 m。可以看出,湿度较大地区的风机雷电屏蔽能力会有所提高,从雷电防护的角度而言,风机间距可以增大;而高海拔地区的风机由于受到空气密度和湿度的共同影响,其雷电屏蔽效应会显著降低。

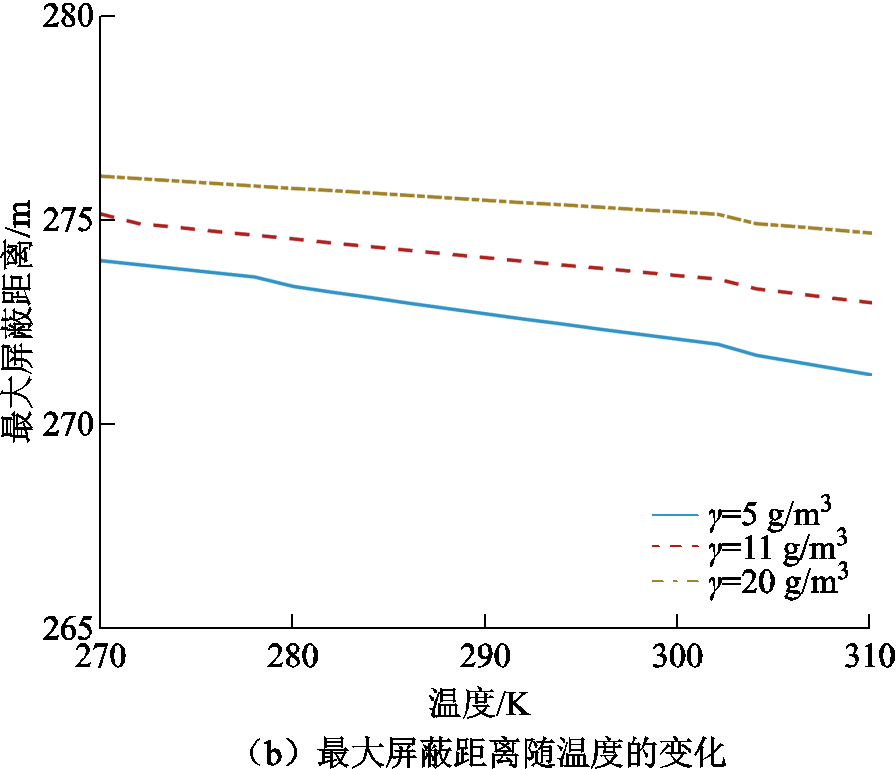
图11 最大屏蔽距离随气压、温度、空气湿度的变化
Fig.11 Variation of maximum shielding distance with changes in atmospheric pressure, temperature, and air humidity
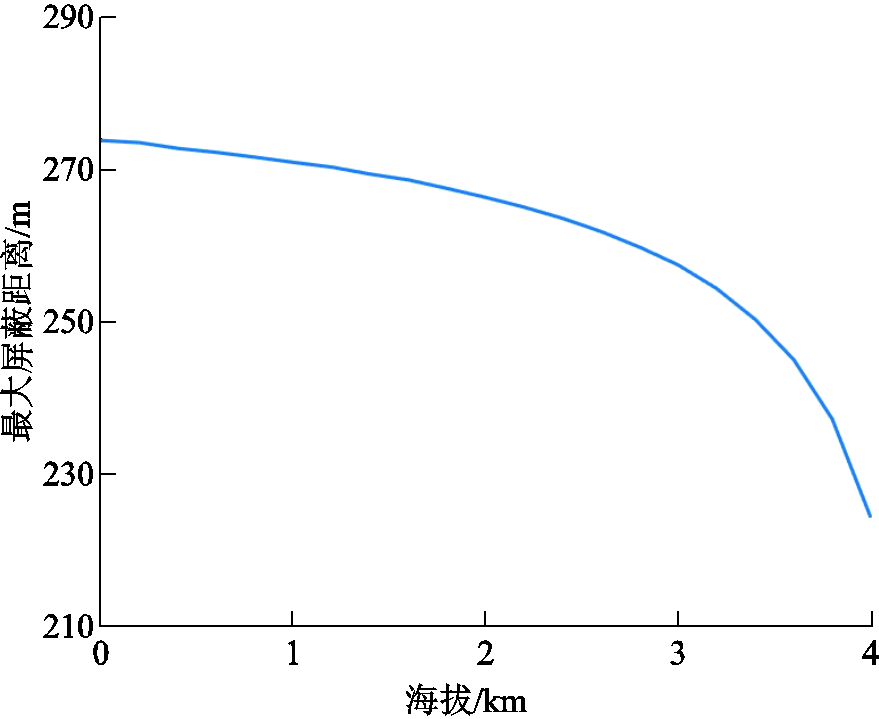
图12 最大屏蔽距离随海拔的变化
Fig.12 Variation of maximum shielding distance with altitude
雷电屏蔽距离的大小会受到上行先导发展的影响,起始于接闪器的上行先导发展越好,证明其对周围环境雷电屏蔽的效果越好。以往利用避雷针对建筑物等易于遭受雷击的高大结构进行保护时,通常仅使用“滚球法”或是电气几何法等不考虑上行先导发展的方法,缺乏物理依据。而最近基于物理场仿真的计算结果表明[30],考虑上行先导发展的雷电屏蔽范围对于复杂地形和复杂结构也能够很好地适应。在本文考虑的高海拔地区或是湿度较大地区,可以依据过去文献得出的公式对模型进行修改进而进行计算分析,能够有效地解决户外难以开展试验等弊端。
本文讨论了环境因素对上行先导的影响,所采用的物理模型属于简化模型,无法对先导的完整物理参数进行详细建模,因此需要与其他试验和仿真结果进行对比。在长气隙放电试验中,He Hengxin等[43]在海拔4 300 m的西藏和海拔53 m的北京进行放电试验,发现正极性先导的发展速度会随着气压的降低而降低。由于随着气体密度的降低,形成初始流注的半径增大,会延缓先导段的加热,从而影响上行先导的后续发展,与本文计算结果趋势相同。
同样地,湿度对先导形成的影响也得到了广泛研究。电晕放电是先导放电的前提,而碰撞电离系数α和吸附系数η是影响这一过程的关键,通过文献[44-45]等的理论和试验分析,发现湿度对空气中电子的碰撞电离系数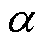 和吸附系数
和吸附系数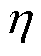 存在以下关系,有
存在以下关系,有
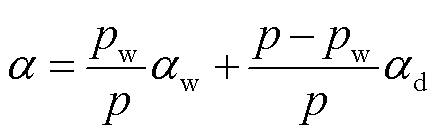 (17)
(17)
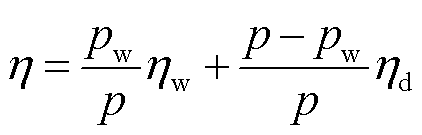 (18)
(18)
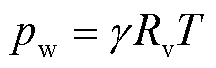 (19)
(19)
式中,pw为水蒸气分压,kPa;αw和αd分别为饱和水蒸气和干燥空气中的碰撞电离系数;ηw和ηd分别为饱和水蒸气和干燥空气中的吸附系数;Rv为水蒸气的比气体常数,在标准大气压下取Rv=461.9 J/(kg·K)。通过计算得到碰撞电离系数和吸附系数随湿度的变化趋势如图13所示。

图13 碰撞电离系数α和吸附系数η随湿度的变化趋势
Fig.13 Variation trends of the collision ionization coefficientα and attachment coefficient η with changes in humidity
可以看出,在电场强度E较小时,随着电场强度的增大,有效电离系数会变小;当环境电场强度大于某一临界值后,碰撞电离系数会随着电场强度的增大而急剧增大,同时吸附系数会随着电场强度的增大而减小。随着湿度增大,碰撞电离系数和吸附系数均会增大,但是在高电场下,碰撞电离系数受到电场的影响更大,因此湿度越大越有利于放电的发生,这与Li Bingxu等通过化学方案计算得出的结果一致[46]。然而湿度大并不能完全反映海上风电场的情形,海上拥有更加复杂的气象,同时海水的盐分可能也会对计算结果产生影响,但基于湿度大的层面,需要考虑其会对周围产生更好的雷电屏蔽效应。
上行先导的竞争机制在一些文献中被提及[9, 23],说明上行先导在决定雷击点时存在至关重要的作用,而上行先导的发展可能就取决于这些环境参数和气象参数,有关上行先导的竞争机制值得进一步研究。
本文利用上行先导起始及发展模型,对在考虑上行先导情况下的风力发电机进行闪击距离的计算,并根据闪击距离对风力发电机的雷电屏蔽效应进行了研究,得出以下结论:
1)随着下行先导电流的增大,风机叶尖接闪器对雷电的吸引范围会变大,意味着随着下行先导电流的增大,上行先导会更早起始,随即得到更好的发展,但是不会成比例增加。因为当回击电流增大时,上、下行先导的击穿会更早发生。
2)随着风机旋转角度的增大,闪击距离也会增大。旋转角度的变化影响风机叶尖接闪器与地面的距离,进而影响电势的分布。旋转角度越大,电势也越大,上行先导越早起始。当旋转角度由90°变为60°时,尖端与地面的距离变化不大,从而影响较小;而旋转角度由60°变化为30°时,其影响较大。
3)随着下行先导偏移,闪击距离也会发生变化。当偏移距离较小时,闪击距离的变化不大;而偏移距离较大时,闪击距离会变大。这是因为随着下行先导的偏移,给予了上行先导更长的发展时间。
4)当两风机之间的距离小于水平屏蔽距离的2倍时,风机之间会产生互相屏蔽效应。通过计算得知,旋转角度为90°时对周围的屏蔽效果最差。
5)大气压强和湿度的增加使得雷电屏蔽效应增大;而海拔的增加会使得雷电屏蔽效应降低。气压与湿度的变化影响碰撞电离和吸附的过程,进而影响先导形成过程中的热过程,因此风电场雷电防护布置应该考虑环境因素的影响。高海拔地区由于气压和温度较低,其雷电屏蔽距离较小,此时可以减小间距以降低因雷击造成的损耗;而在湿度较大地区,因为其雷电屏蔽距离较大,可以增加风机间距。
参考文献
[1] 朱玲, 李威, 王骞, 等. 基于校正条件生成对抗网络的风电场群绿氢储能系统容量配置[J]. 电工技术学报, 2024, 39(3): 714-730.
Zhu Ling, Li Wei, Wang Qian, et al. Wind farms-green hydrogen energy storage system capacity sizing method based on corrected-conditional generative adversarial network[J]. Transactions of China Electrotechnical Society, 2024, 39(3): 714-730.
[2] 胡琴, 王欢, 舒立春, 等. 覆冰条件下风力发电机叶片防/除冰方法综述[J]. 电工技术学报, 2024, 39(17): 5482-5496.
Hu Qin, Wang Huan, Shu Lichun, et al. Review of anti-/de-icing methods for wind turbine blades under icing conditions[J]. Transactions of China Electro-technical Society, 2024, 39(17): 5482-5496.
[3] Radičević B M, Savić M S, Madsen S F, et al. Impact of wind turbine blade rotation on the lightning strike incidence–a theoretical and experimental study using a reduced-size model[J]. Energy, 2012, 45(1): 644-654.
[4] 蔡国伟, 雷宇航, 葛维春, 等. 高寒地区风电机组雷电防护研究综述[J]. 电工技术学报, 2019, 34(22): 4804-4815.
Cai Guowei, Lei Yuhang, Ge Weichun, et al. Review of research on lightning protection for wind turbines inalpine areas[J]. Transactions of China Electrotechnical Society, 2019, 34(22): 4804-4815.
[5] 黄怡鋆, 樊亚东, 王红斌, 等. 组合八邻域跟踪算法监测全闪电雷暴活动时空演变过程及特征[J]. 电工技术学报, 2024, 39(5): 1536-1547.
Huang Yijun, Fan Yadong, Wang Hongbin, et al. Spatial-temporal evolution process and characteristics of thunderstorm activity based on combinatorial eight-connectivity tracking algorithm[J]. Transactions of China Electrotechnical Society, 2024, 39(5): 1536-1547.
[6] 蔡力, 田汭鑫, 魏俊涛, 等. 连续冲击电流脉冲下避雷器阀片电气性能研究[J]. 电工技术学报, 2023, 38(增刊1): 168-176.
Cai Li, Tian Ruixin, Wei Juntao, et al. Research on the electrical performance of ZnO varistors under multiple impulse current pulse[J]. Transactions of China Electrotechnical Society, 2023, 38(S1): 168-176.
[7] 蔡力, 杜懿阳, 胡强, 等. 火箭引雷至架空线路与地面电流对比分析[J]. 电工技术学报, 2024, 39(3): 914-923.
Cai Li, Du Yiyang, Hu Qiang, et al. Comparative analysis of current of rocket-triggered lightning striking the overhead line and the ground[J]. Transactions of China Electrotechnical Society, 2024, 39(3): 914-923.
[8] 蔡力, 杜懿阳, 胡强, 等. 火箭引雷至架空线路与地面近距离磁场对比分析[J]. 电工技术学报, 2023, 38(24): 6798-6806.
Cai Li, Du Yiyang, Hu Qiang, et al. Comparative analysis of close magnetic field of rocket-triggered lightning striking the overhead line and the ground[J]. Transactions of China Electrotechnical Society, 2023, 38(24): 6798-6806.
[9] 李庆民, 郭子炘, 张黎, 等. 大型风电场雷击防护研究面临的关键问题[J]. 中国电机工程学报, 2018, 38(18): 5296-5306.
Li Qingmin, Guo Zixin, Zhang Li, et al. Key issues with lightning protection research of the large-scale wind farms[J]. Proceedings of the CSEE, 2018, 38(18): 5296-5306.
[10] 蔡力, 柯逸丰, 李进, 等. 基于高速摄像观测的风电场雷击风机发展过程和特性分析[J]. 电工技术学报, 2021, 36(增刊1): 303-310.
Cai Li, Ke Yifeng, Li Jin, et al. Development process and characteristic analysis of the natural lightning strike on wind turbine based on high-speed camera observation[J]. Transactions of China Electrotechnical Society, 2021, 36(S1): 303-310.
[11] 任瀚文, 郭子炘, 马宇飞, 等. 雷击风机叶片的跃变击距特性与定量表征[J]. 电工技术学报, 2017, 32(15): 216-224.
Ren Hanwen, Guo Zixin, Ma Yufei, et al. Quantitative characterization of the striking saltus distance of wind turbine blade[J]. Transactions of China Electrotechnical Society, 2017, 32(15): 216-224.
[12] Zhou Qibin, Liu Canxiang, Bian Xiaoyan, et al. Numerical analysis of lightning attachment to wind turbine blade[J]. Renewable Energy, 2018, 116: 584-593.
[13] 张萍, 赵新贺, 聂鑫鹏, 等. 基于ATP-EMTP的风电机组塔筒二次雷电回击暂态分析[J]. 高压电器, 2023, 59(5): 163-169.
Zhang Ping, Zhao Xinhe, Nie Xinpeng, et al. Transient analysis of secondary lightning strike in wind turbine tower based on ATP-EMTP[J]. High Voltage Apparatus, 2023, 59(5): 163-169.
[14] Guo Zixin, Li Qingmin, Ma Yufei, et al. Experimental study on lightning attachment manner to wind turbine blades with lightning protection system[J]. IEEE Transactions on Plasma Science, 2019, 47(1): 635-646.
[15] 赵泽洋, 肖慈恩, 刘亚坤, 等. 接闪阳极参数对雷电弧材料损伤数值分析的影响[J]. 电工技术学报, 2024, 39(5): 1486-1496.
Zhao Zeyang, Xiao Cien, Liu Yakun, et al. Influence of anode on numerical analysis of arc-material interactions with multi-field coupling in lightning damage[J]. Transactions of China Electrotechnical Society, 2024, 39(5): 1486-1496.
[16] He Jinliang, Yang Guohua, Li Quanxin, et al. First altitude-triggered lightning experiment associated with an elevated wind turbine blade on the ground[J]. Geophysical Research Letters, 2024, 51(18): e2024GL109400.
[17] 文习山, 邓冶强, 王羽, 等. 风力发电机叶片雷击接闪特性研究综述[J]. 高电压技术, 2020, 46(7): 2511-2521.
Wen Xishan, Deng Yeqiang, Wang Yu, et al. Review of research on lightning striking performance of wind turbine blade[J]. High Voltage Engineering, 2020, 46(7): 2511-2521.
[18] Rachidi F, Rubinstein M, Montanya J, et al. A review of current issues in lightning protection of new-generation wind-turbine blades[J]. IEEE Transactions on Industrial Electronics, 2008, 55(6): 2489-2496.
[19] Dwyer J R, Uman M A. The physics of lightning[J]. Physics Reports, 2014, 534(4): 147-241.
[20] Warner T A, Helsdon J H, Bunkers M J, et al. UPLIGHTS: upward lightning triggering study[J]. Bulletin of the American Meteorological Society, 2013, 94(5): 631-635.
[21] Wang D, Takagi N, Watanabe T, et al. Observed characteristics of upward leaders that are initiated from a windmill and its lightning protection tower[J]. Geophysical Research Letters, 2008, 35(2): L0280.
[22] Saba M M F, Paiva A R, Schumann C, et al. Lightning attachment process to common buildings[J]. Geophysical Research Letters, 2017, 44(9): 4368-4375.
[23] Cai Li, Ke Yifeng, Fan Wenchao, et al. Observation of an upward lightning flash with 21 upward positive leaders initiated from different wind turbines in wind farm[J]. High Voltage, 2024, 9(1): 163-171.
[24] Candela Garolera A, Cummins K L, Madsen S F, et al. Multiple lightning discharges in wind turbines associated with nearby cloud-to-ground lightning[J]. IEEE Transactions on Sustainable Energy, 2015, 6(2): 526-533.
[25] Ma Ziwei, Jasni J, Ab Kadir M Z A, et al. Numerical model of lightning attachment on UHV-AC trans-mission lines and effects of operating voltage, phase angle, and terrain[J]. Electric Power Systems Research, 2024, 227: 109986.
[26] Tavakoli M R B, Vahidi B. Transmission-lines shielding failure-rate calculation by means of 3-D leader progression models[J]. IEEE Transactions on Power Delivery, 2011, 26(2): 507-516.
[27] 雷宇航, 蔡国伟, 潘超. 大气条件下雷击风机叶片初始流注区电场强度与临界长度研究[J]. 电工技术学报, 2019, 34(20): 4392-4399.
Lei Yuhang, Cai Guowei, Pan Chao. Research of electric field of lightning initial streamer from wind turbine blade and critical length based on atmospheric conditions[J]. Transactions of China Electrotechnical Society, 2019, 34(20): 4392-4399.
[28] Bian Xiaoyan, Wu Yong, Zhou Qibin, et al. Quantitative characteristics of the striking distance to wind turbine blades based on an improved stochastic lightning model[J]. IET Generation, Transmission & Distribution, 2023, 17(10): 2317-2330.
[29] 黄胜鑫, 陈维江, 贺恒鑫, 等. 风力发电机组下行雷击风险计算方法[J]. 中国电机工程学报, 2019, 39(12): 3541-3551.
Huang Shengxin, Chen Weijiang, He Hengxin, et al. Downward lightning risk assessment method asso-ciated with wind turbine[J]. Proceedings of the CSEE, 2019, 39(12): 3541-3551.
[30] Nie Jiayi, Xiang Nianwen, Li Kejie, et al. Multiple wind turbines shielding model of lightning attractiveness for mountain wind farms[J]. Electric Power Systems Research, 2023, 224: 109727.
[31] Zhang Li, Wang Guozheng, Zhang Wenfang, et al. An electro-geometric model for lightning shielding of multiple wind turbines[J]. Energies, 2017, 10(9): 1272.
[32] Yang Ning, Zhang Qilin, Hou Wenhao, et al. Analysis of the lightning-attractive radius for wind turbines considering the developing process of positive atta-chment leader[J]. Journal of Geophysical Research: Atmospheres, 2017, 122(6): 3481-3491.
[33] Cooray V, Rakov V, Theethayi N. The lightning striking distance—revisited[J]. Journal of Electrostatics, 2007, 65(5/6): 296-306.
[34] Becerra M, Cooray V. A simplified physical model to determine the lightning upward connecting leader inception[J]. IEEE Transactions on Power Delivery, 2006, 21(2): 897-908.
[35] Becerra M, Cooray V. Time dependent evaluation of the lightning upward connecting leader inception[J]. Journal of Physics D: Applied Physics, 2006, 39(21): 4695-4702.
[36] Rizk F A M. A model for switching impulse leader inception and breakdown of long air-gaps[J]. IEEE Transactions on Power Delivery, 1989, 4(1): 596-606.
[37] Lalande P, Bondiou-Clergerie A, Bacchiega G, et al. Observations and modeling of lightning leaders[J]. Comptes Rendus Physique, 2002, 3(10): 1375-1392.
[38] Dellera L, Garbagnati E. Lightning stroke simulation by means of the leader progression model. I. Description of the model and evaluation of exposure of free-standing structures[J]. IEEE Transactions on Power Delivery, 1990, 5(4): 2009-2022.
[39] Candela Garolera A, Madsen S F, Nissim M, et al. Lightning damage to wind TurbineBlades from wind farms in the U.S[J]. IEEE Transactions on Power Delivery, 2016, 31(3): 1043-1049.
[40] Becerra M. Corona discharges and their effect on lightning attachment revisited: upward leader initiation and downward leader interception[J]. Atmospheric Research, 2014, 149: 316-323.
[41] Eriksson A J, le Roux B C, Geldenhuys H J, et al. Study of airgap breakdown characteristics under ambient conditions of reduced air density[J]. IEE Proceedings A Physical Science, Measurement and Instrumentation, Management and Education, Reviews, 1986, 133(8): 485.
[42] Petrov N I, Waters R T. Determination of the striking distance of lightning to earthed structures[J]. Procee-dings of the Royal Society of London Series A: Mathematical and Physical Sciences, 1995, 450(1940): 589-601.
[43] He Hengxin, Liu Lipeng, Ding Yujian, et al. Positive leaders propagate slower at higher altitudes: experimental evidence and theoretical explanation[J]. Geophysical Research Letters, 2022, 49(4): e2021GL095995.
[44] Abdel-Salam M. Positive wire-to-plane coronas as influenced by atmospheric humidity[J]. IEEE Transactions on Industry Applications, 1985, IA-21(1): 35-40.
[45] Bian Xingming, Wang Liming, MacAlpine J K, et al. Positive corona inception voltages and corona currents for air at various pressures and humidities[J]. IEEE Transactions on Dielectrics and Electrical Insulation, 2010, 17(1): 63-70.
[46] Li Bingxu, Li Xingwen, Fu Mingli, et al. Effect of humidity on dielectric breakdown properties of air considering ion kinetics[J]. Journal of Physics D Applied Physics, 2018, 51(37): 375201.
Investigation of Lightning Shielding Distances Between Adjacent Wind Turbines Considering Upward Leader Development
Abstract Wind power is a rapidly developing renewable energy source; however, the unique size and location of wind turbines pose significant lightning threats to the safe and stable operation of wind farms. Unlike traditional stationary structures, wind turbines are more prone to initiating upward leaders at blade tips due to their rotational characteristics. Consequently, previous lightning protection models that neglected upward leaders may not be suitable for evaluating lightning protection in wind turbines. This study aims to establish a simulation model for wind turbines, utilizing upward and downward leader propagation models to compute the striking distance and analyze the lightning shielding effects and influencing factors in wind farms.
A full-scale 3 MW wind turbine model is established with defined downward leader charge distribution, propagation speed, thundercloud charges, and simulation boundaries. The initiation and propagation of upward leaders are calculated and analyzed using a self-consistent leader inception and propagation model. Geometric factor is introduced to linearize the potential distribution in the streamer region to simplify computations. The simulation accounts for the effects of downward leader propagation, upward leader initiation and propagation, and leader connection.
Results show that striking distances vary with downward leader offsets and blade rotation angles, as different scenarios affect upward leader propagation. Longer development times improve upward leader propagation, requiring more time for breakdown between upward and downward leaders when offsets occur. Larger blade rotation angles increase potential gradients at blade tips, leading to earlier upward leader initiation and longer development.
Lightning shielding effects are analyzed based on striking distances. Shielding is weakest at a 90-degree blade rotation angle and slightly better at 30-degrees blade rotation angle. Environmental parameters, including atmospheric pressure and humidity, enhance shielding, while altitude reduces it. These findings highlight the importance of considering environmental factors in lightning protection designs for wind turbines.
Keywords:Wind turbine clusters, upward leader, lightning protection, shielding effect, environmental parameter analysis
中图分类号:TM865
DOI: 10.19595/j.cnki.1000-6753.tces.241925
国家自然科学基金(52177154)和湖北省自然科学基金杰出青年基金(2024AFA060)资助项目。
收稿日期 2024-10-28
改稿日期 2024-11-25
蔡 力 男,1987年生,教授,博士生导师,研究方向为雷电物理和雷电防护。
E-mail:caili@whu.edu.cn
樊文超 男,2001年生,博士研究生,研究方向为新能源雷电防护。
E-mail:2279878803@qq.com(通信作者)
(编辑 李 冰)