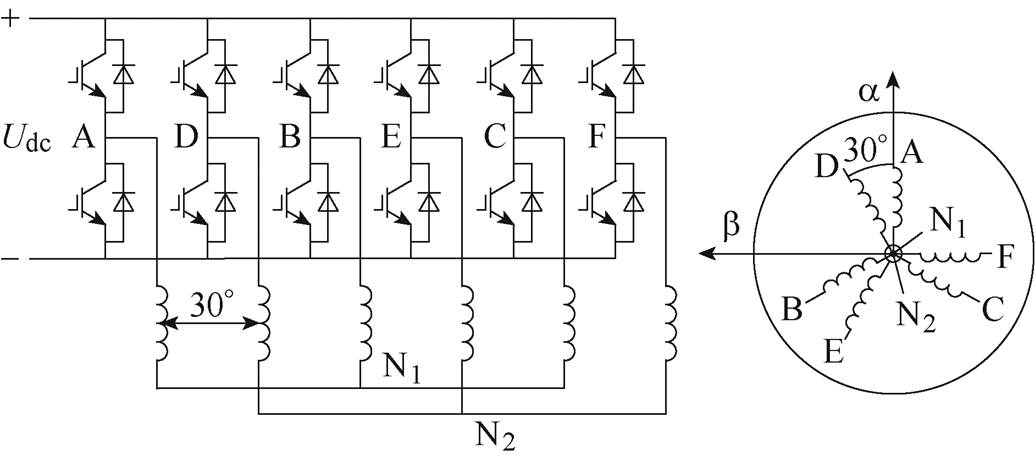
图1 双三相电机驱动系统
Fig.1 Dual three-phase motor drive system
摘要 正常双三相电机直接转矩控制运行需要对基本电压矢量进行修正,采用修正后的虚拟矢量进行直接转矩控制可以抑制谐波电流。双三相电机缺一相故障直接转矩控制时,需要对电压矢量重新进行修正。该文给出了双三相电机缺一相故障情况下的电压矢量分布,提出一种双三相电机缺一相运行时虚拟矢量的间接修正方法,并且使虚拟矢量的幅值达到最大。构建双三相电机缺一相容错直接转矩控制策略,其定子磁链分区和开关表的选取规则与双三相电机正常运行时一致,其差别只是缺一相运行时十二个虚拟矢量的幅值有所减少,因此比较易于实现。实验结果验证了所提方法的正确性和可行性。
关键词:双三相永磁同步电机 直接转矩控制 单相开路故障 虚拟矢量修正
近年来,多相电机驱动系统因其具有低压大功率输出和高可靠性等优势,在航空航天、舰船电力推进、电动汽车等领域具有广泛的应用前景[1-4]。在各类多相电机中,六相电机由于和传统三相电机具有紧密的联系而得到了更多的关注。其中相移30°双三相电机(即不对称六相电机)消除了6次谐波转矩脉动,其在抑制转矩脉动上具有更大的优势,因此得到了广泛的研究[5-10]。
与传统三相电机类似,直接转矩控制(Direct Torque Control, DTC)因其结构简单、响应快、参数依赖性小等优点,也被广泛应用于多相电机控制中。为了抑制多相电机谐波子平面的谐波电流,国内外学者提出了基于虚拟矢量的DTC算法,即在一个控制周期内将基本电压矢量按照特定比例合成虚拟矢量,使虚拟矢量在谐波子平面的电压为零,采用虚拟矢量构建开关表对多相电机进行直接转矩控制[11-18]。文献[18]在控制周期中插入零矢量,通过优化虚拟电压矢量占空比以减小转矩脉动。文献[19]针对双三相永磁同步电机(Permanent Magnet Syn- chronous Motor, PMSM)提出了一种新颖的混合DTC算法,在电机的稳态和动态两种模式下分别用不同的转矩控制方法,实现平滑过渡的同时电机驱动系统具有良好的动静态性能。
对于多相电机而言,适于容错运行是它的一个显著优势。电机驱动系统中的各种开路和短路故障通过故障隔离的方法都可以转化为电机的缺相运行。当多相电机发生单相开路故障缺相运行时,不需要改动任何硬件拓扑,只需选择适当的容错控制策略便可降额继续运行,避免了停机或系统重组。正常多相电机直接转矩控制运行时需要对电压矢量进行修正,当多相电机发生缺相故障时,同样需要对缺相后的电压矢量进行修正。文献[20-21]针对五相电机单相故障提出了一种基于虚拟矢量的DTC方法,构建了五相电机故障后的八个虚拟矢量,并重新构建了故障后的开关表,采用虚拟矢量的DTC可降低计算量,且具有较强的鲁棒性,有效抑制了3次谐波电流和转矩脉动。文献[22]针对偏置60°六相对称PMSM发生单相绕组开路故障,提出了容错型DTC策略,采用重构虚拟变量、虚拟定子电压矢量方法,实现圆形虚拟定子磁链轨迹控制,进而实现电磁转矩直接控制。虚拟矢量同样可以应用到多相电机的空间矢量脉宽调制(Space Vector Pulse Width Modulation, SVPWM)和预测电流控制中,文献[23]构建五相电机故障后的虚拟矢量进行SVPWM,以实现缺相后的容错矢量控制。文献[24-25]将五相电机故障后的虚拟矢量应用到缺相后的有限集模型预测控制中。上述基于合成虚拟矢量进行控制的缺相容错DTC策略,存在一个共同问题,就是电机在发生故障后,虚拟矢量的空间相位发生变化,需重新进行定子磁链分区和开关表的设计,使得控制过程,尤其是正常运行到缺相容错运行的切换较为复杂。
本文以发生单相开路故障的相移30°双三相PMSM为研究对象,提出了一种双三相电机缺相容错运行虚拟电压矢量的间接修正方法,并且构建了基于虚拟矢量的无需重新设计定子磁链分区和开关表的容错型DTC策略。首先给出了双三相PMSM正常和单相开路故障下的电压矢量分布。阐述了正常情况下基于虚拟矢量的DTC基本原理,然后研究了双三相PMSM缺一相故障运行时虚拟矢量的修正方法,并且消除了虚拟矢量中包含的零矢量,实现了最大直流母线电压利用。实验结果表明所提容错型DTC算法有效地抑制了缺相故障运行时的转矩脉动,并且具有良好的稳态和动态性能。
相移30°双三相电机驱动系统如图1所示。根据矢量空间解耦理论,通过静止坐标变换式(1),双三相电机的所有变量被映射到ab、z1z2和o1o2三个相互正交的子平面中,其中ab 子平面参与机电能量转换。o1o2子平面为零序子平面。在中性点相互隔离的情况下,对应到o1o2子平面中的变量都是零。

图1 双三相电机驱动系统
Fig.1 Dual three-phase motor drive system
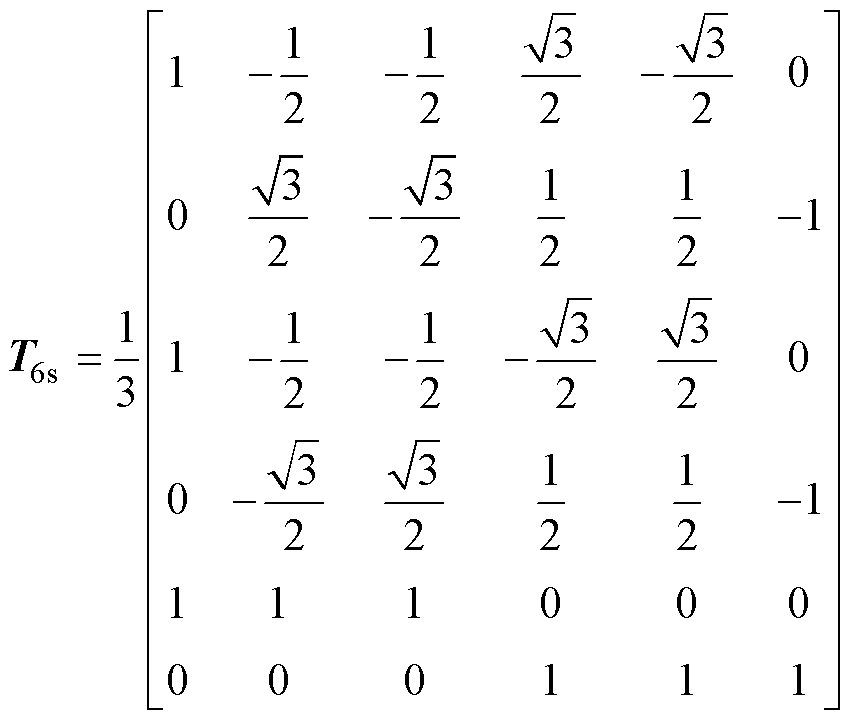 (1)
(1)
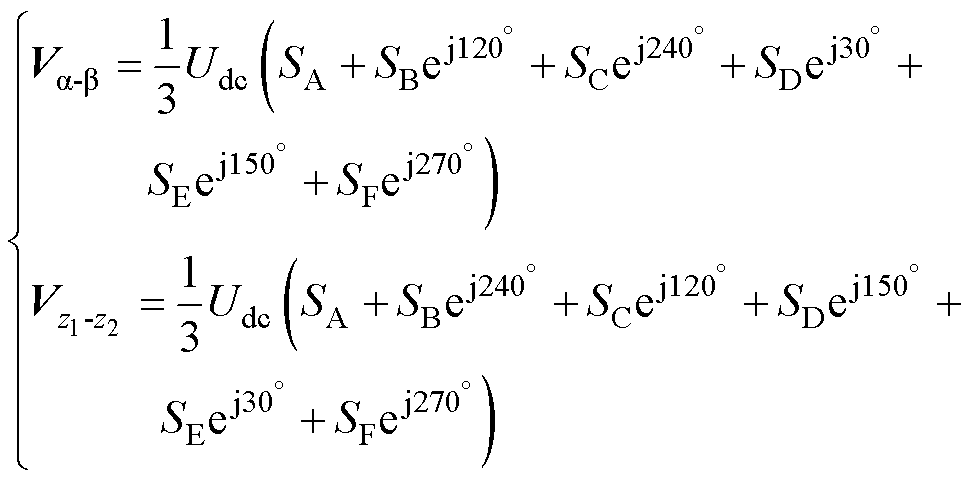 (2)
(2)
应用式(2)将电压矢量映射到ab 和z1z2这两个正交子平面,设六相逆变器的开关函数为S=[SASB SC SD SE SF],以A相为例,若SA=1,则表示逆变器A相桥臂上开关导通;SA=0表示A相桥臂下开关导通。从高位到低位按照SASBSCSDSESF的顺序对64个基本电压矢量用八进制数表示,包括4个零矢量和60个有效矢量。由图2所示,将电压矢量根据幅值大小共分为四组,分别为大矢量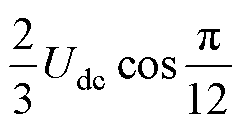 、中矢量
、中矢量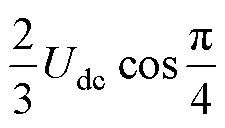 、原始矢量
、原始矢量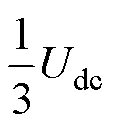 和小矢量
和小矢量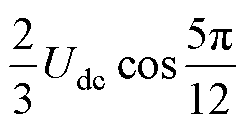 。
。
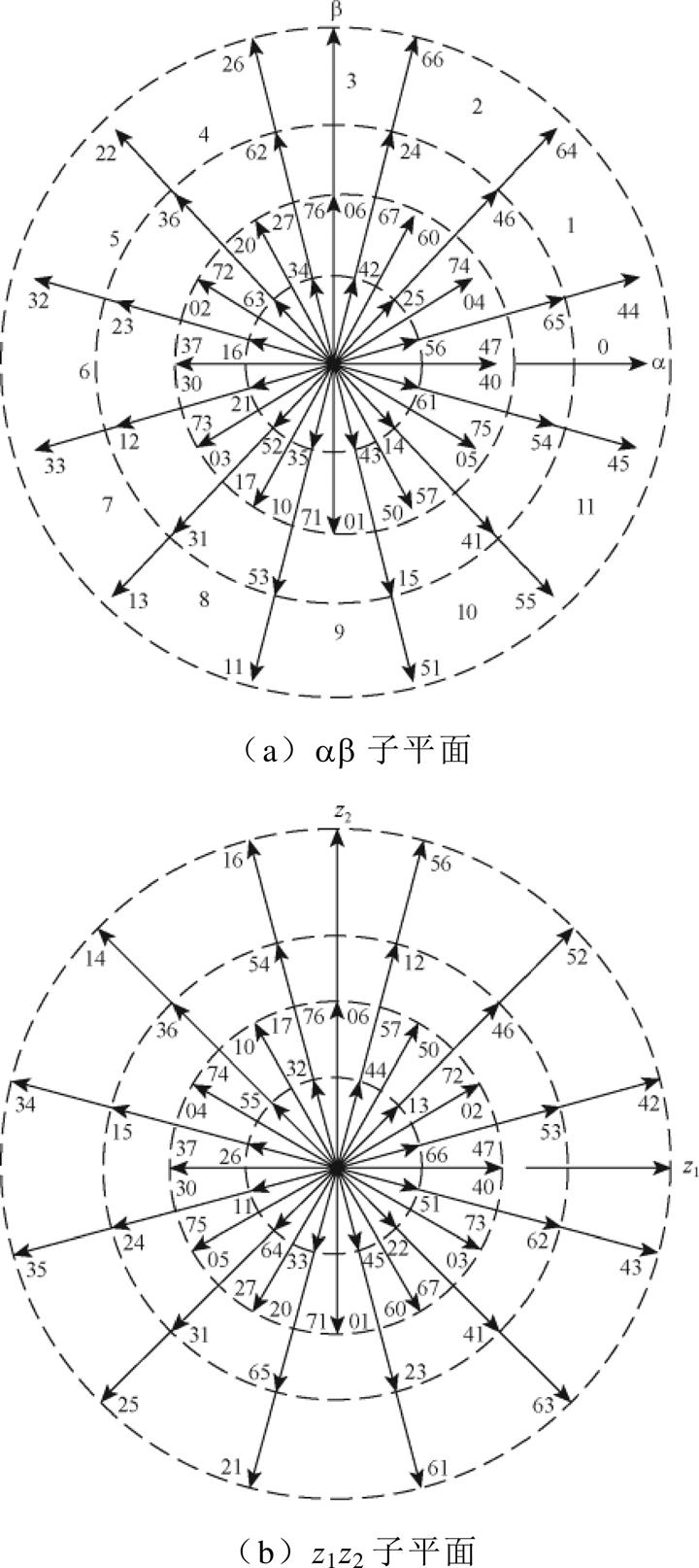
图2 正常运行时ab 和z1z2子平面电压矢量
Fig.2 Voltage vectors in the ab and z1z2 sub-planes during the healthy operation
当双三相PMSM驱动系统发生单相开路故障时(以F相故障为例),此时系统主电路拓扑如图3所示(中性点隔离)。双三相PMSM一相开路故障后,由于缺少了一个桥臂,基本电压矢量由原来的64个变为32个,一相开路故障后的32个基本电压矢量按照SASBSCSDSE(二进制)的顺序重新表示为V00~V31。其中包含28个有效矢量和4个零矢量。双三相PMSM发生F相开路故障后剩余各相电压可以表示为
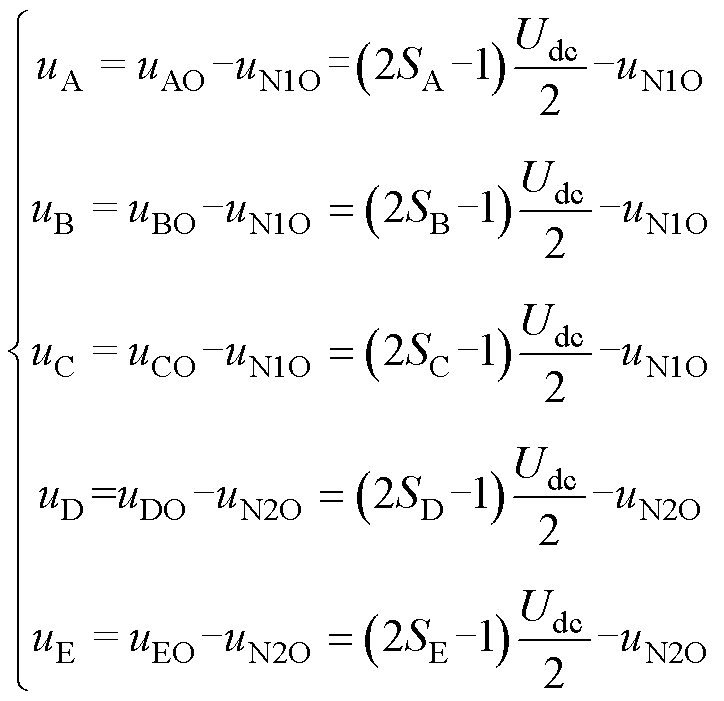 (3)
(3)
式中,uA、uB、uC、uD、uE分别为各相相电压;uAO、uBO、uCO、uDO、uEO分别为各相对应的极电压(逆变器输出端对母线中点);uN1O、uN2O分别为双三相电机两个隔离中性点相对直流母线中点的电压。
双三相PMSM发生单相开路故障后,正常的静止坐标变换矩阵(见式(1))将不再适用缺相后的系统,同样根据基波子平面和谐波子平面互相解耦的原则,构建单相开路故障时的静止坐标变换矩阵T5s[26]为
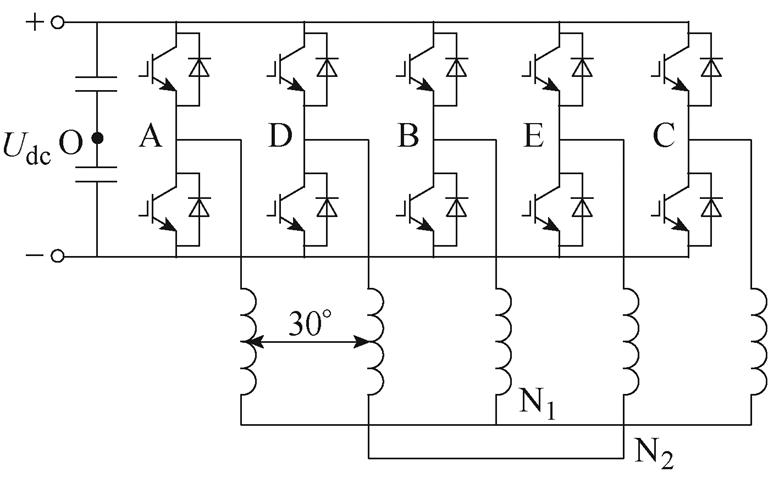
图3 双三相PMSM系统单相开路故障下的电路拓扑
Fig.3 Circuit topology under the open-phase fault of dual three-phase PMSM system
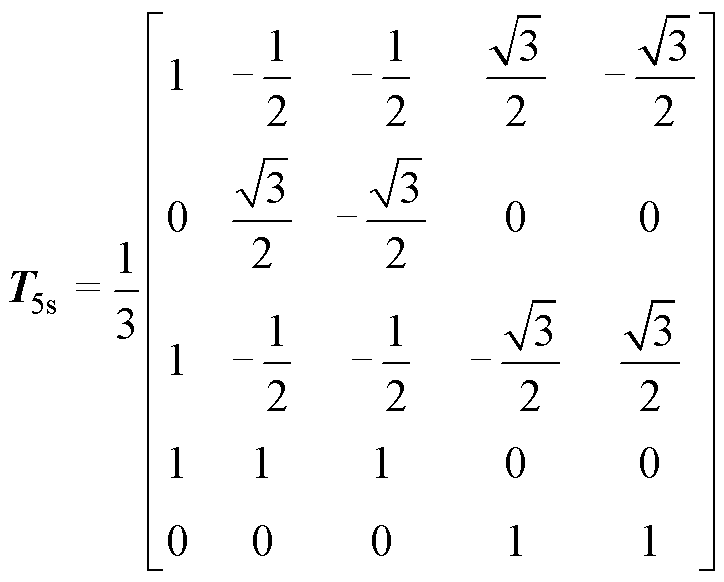 (4)
(4)
对剩余的五相相电压进行静止坐标变换,即
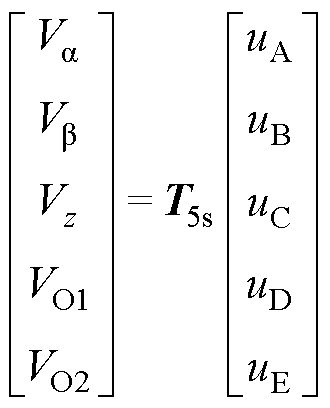 (5)
(5)
将式(3)和式(4)代入式(5)中可以得到
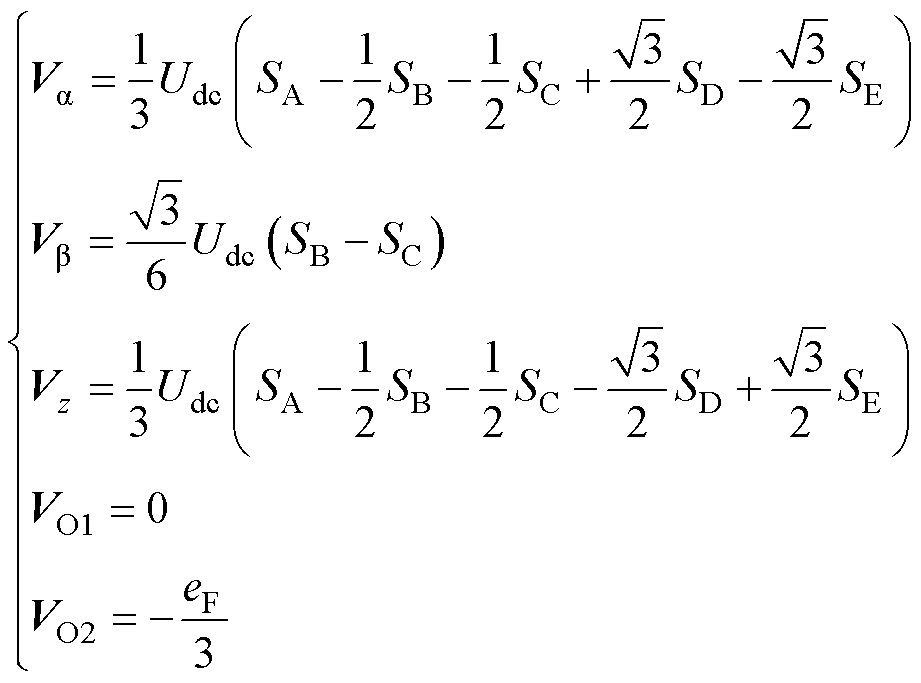 (6)
(6)
式中,eF为故障F相绕组的反电动势。由式(6)所示,当双三相PMSM发生单相开路故障后,系统不再对称,VO2不再为零。根据式(6),可以得到双三相PMSM发生单相开路故障后ab 子平面和z子平面电压矢量如图4所示,具体每个电压矢量的幅值和相位见表1详细列出。
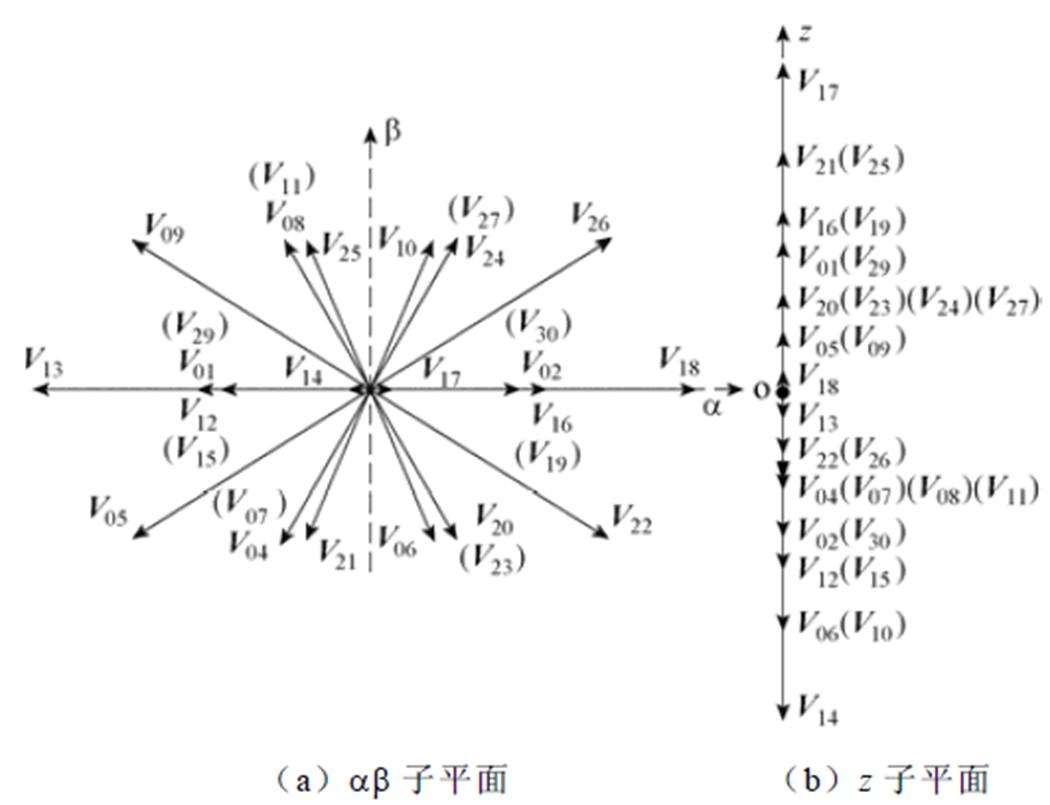
图4 单相故障后ab 子平面和z子平面的电压矢量
Fig.4 Voltage vectors in the ab and z sub-planes in one open-phase fault operation
表1 单相开路故障下ab子平面和z子平面基本电压矢量
Tab.1 Basic voltage vectors in the ab and z sub-planes under one open-phase fault operation
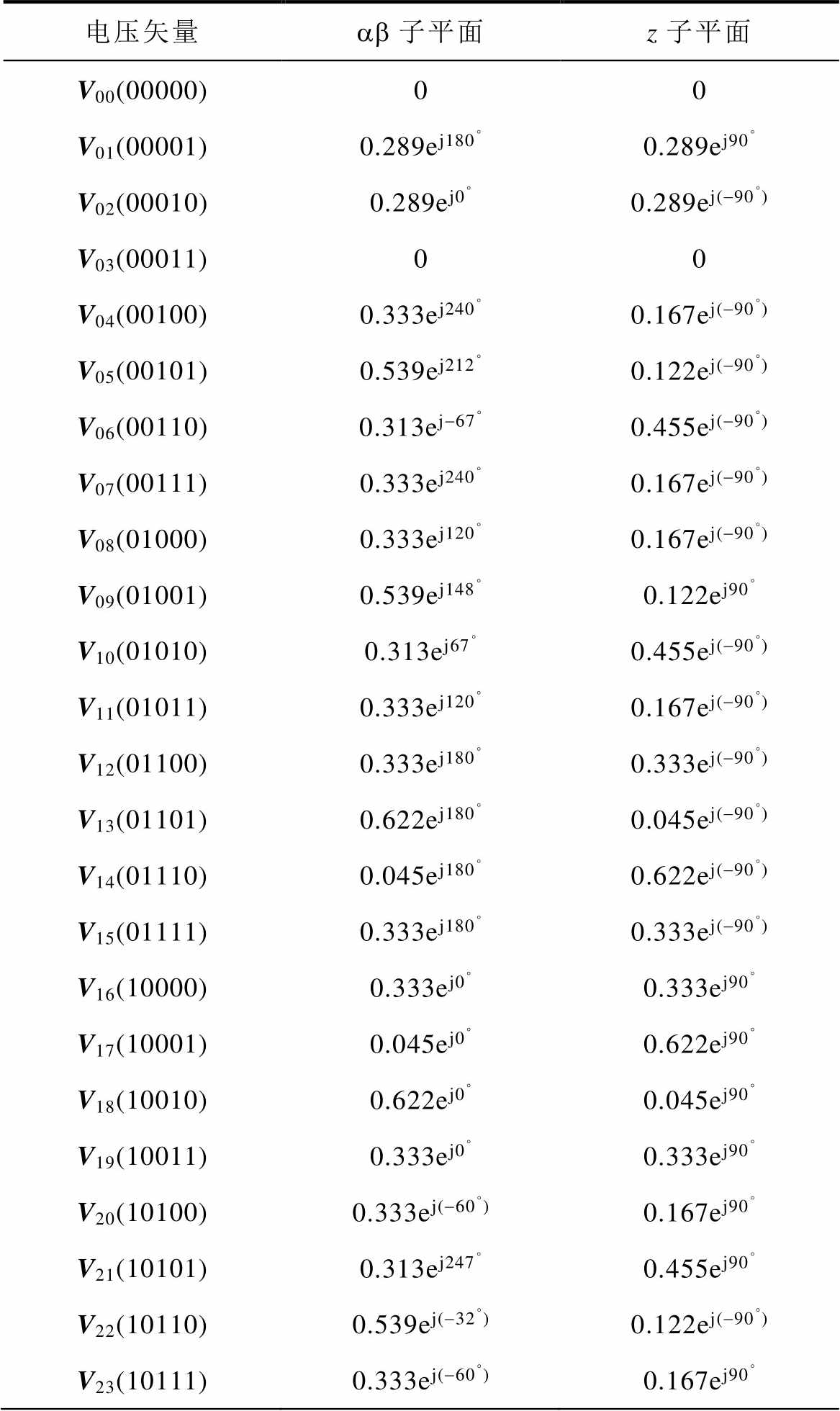
电压矢量ab 子平面z子平面 V00(00000)00 V01(00001)0.289ej180°0.289ej90° V02(00010)0.289ej0°0.289ej(-90°) V03(00011)00 V04(00100)0.333ej240°0.167ej(-90°) V05(00101)0.539ej212°0.122ej(-90°) V06(00110)0.313ej-67°0.455ej(-90°) V07(00111)0.333ej240°0.167ej(-90°) V08(01000)0.333ej120°0.167ej(-90°) V09(01001)0.539ej148°0.122ej90° V10(01010)0.313ej67°0.455ej(-90°) V11(01011)0.333ej120°0.167ej(-90°) V12(01100)0.333ej180°0.333ej(-90°) V13(01101)0.622ej180°0.045ej(-90°) V14(01110)0.045ej180°0.622ej(-90°) V15(01111)0.333ej180°0.333ej(-90°) V16(10000)0.333ej0°0.333ej90° V17(10001)0.045ej0°0.622ej90° V18(10010)0.622ej0°0.045ej90° V19(10011)0.333ej0°0.333ej90° V20(10100)0.333ej(-60°)0.167ej90° V21(10101)0.313ej247°0.455ej90° V22(10110)0.539ej(-32°)0.122ej(-90°) V23(10111)0.333ej(-60°)0.167ej90°
(续)
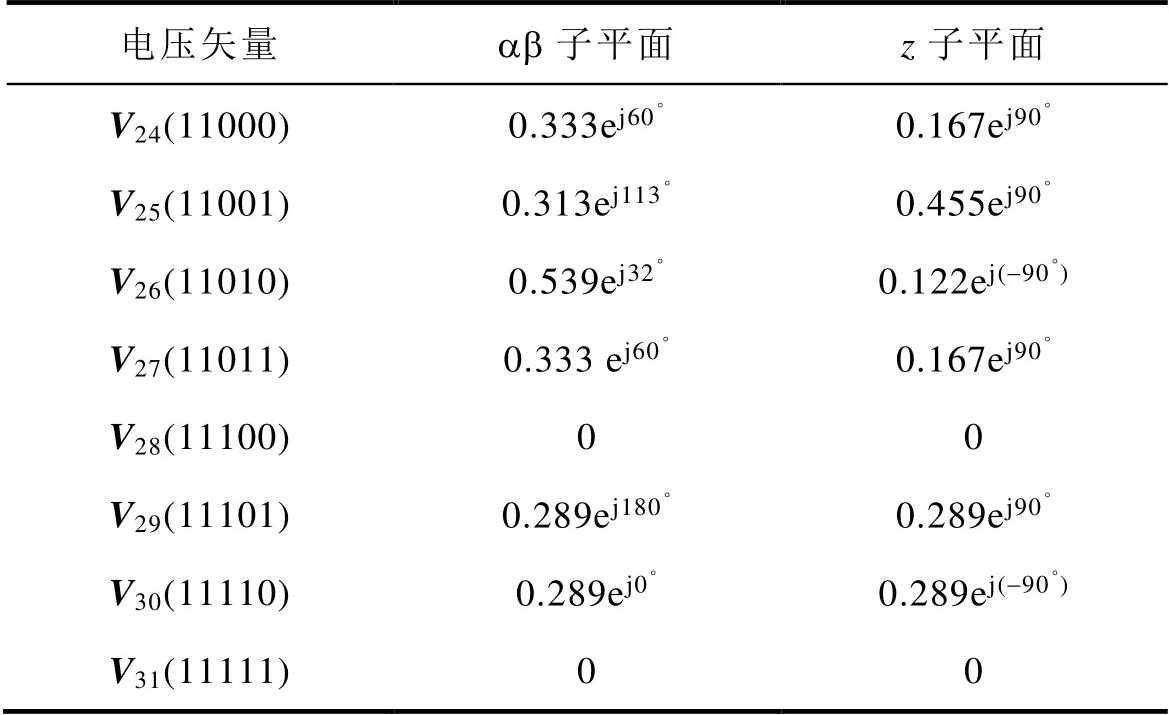
电压矢量ab 子平面z子平面 V24(11000)0.333ej60°0.167ej90° V25(11001)0.313ej113°0.455ej90° V26(11010)0.539ej32°0.122ej(-90°) V27(11011)0.333 ej60°0.167ej90° V28(11100)00 V29(11101)0.289ej180°0.289ej90° V30(11110)0.289ej0°0.289ej(-90°) V31(11111)00
由于双三相电机基本电压矢量在z1z2子平面上的电压矢量并不为0,且z1z2子平面的谐波阻抗仅由定子电阻和漏感组成,很低的谐波电压就可以产生大量的谐波电流,长时间运行会对电机造成损坏。因此双三相电机直接转矩控制不能直接采用基本电压矢量,必须对电压矢量进行修正。
由图2所示,只需控制大矢量和中矢量在一个控制周期之内的作用时间与小矢量及中矢量的幅值成反比关系,即可保证在z1z2子平面上的合成电压矢量为零。定义ab 子平面上同一方向上的大矢量和中矢量合成的新电压矢量为虚拟矢量,设控制周期为Ts,大矢量作用时间为lTs,则中矢量作用时间(1-l)Ts,虚拟矢量在ab 和z1z2子平面的幅值分别为
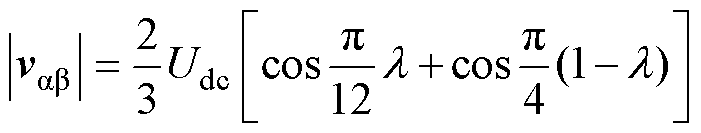 (7)
(7)
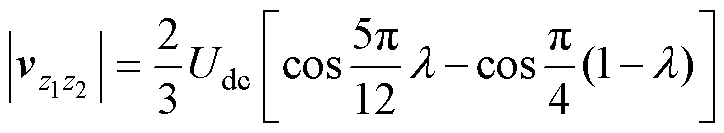 (8)
(8)
可以得到当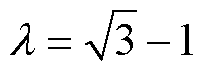 时,
时,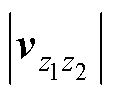 =0,此时
=0,此时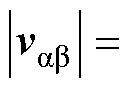
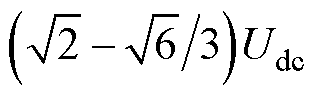 ,即在ab 子平面上合成的虚拟矢量幅值约为0.597 7Udc,如图5所示,图中VL、VM和VV分别表示大矢量、中矢量和虚拟矢量。基于虚拟矢量的DTC算法将ab 子平面分成了12个扇区,可构造如表2所示的开关表。表2中,Fy 和FT分别为定子磁链和电磁转矩控制信号,1表示参考值大于观测值即需要控制该变量增加,0表示参考值小于观测值即需要控制该变量减少。采用修正后的虚拟矢量进行直接转矩控制可以很好地抑制z1z2子平面的谐波电流,从而减少系统损耗。基于虚拟矢量的双三相PMSM直接转矩控制方法详细介绍参见文献[14]。
,即在ab 子平面上合成的虚拟矢量幅值约为0.597 7Udc,如图5所示,图中VL、VM和VV分别表示大矢量、中矢量和虚拟矢量。基于虚拟矢量的DTC算法将ab 子平面分成了12个扇区,可构造如表2所示的开关表。表2中,Fy 和FT分别为定子磁链和电磁转矩控制信号,1表示参考值大于观测值即需要控制该变量增加,0表示参考值小于观测值即需要控制该变量减少。采用修正后的虚拟矢量进行直接转矩控制可以很好地抑制z1z2子平面的谐波电流,从而减少系统损耗。基于虚拟矢量的双三相PMSM直接转矩控制方法详细介绍参见文献[14]。
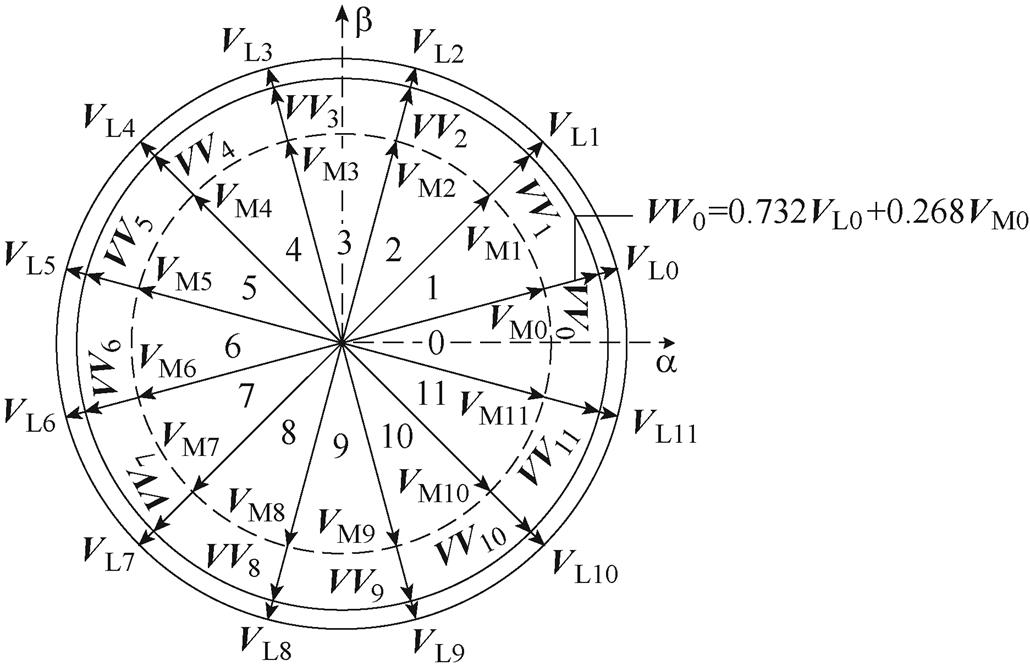
图5 正常运行时ab 子平面中的虚拟矢量
Fig.5 Virtual voltage vectors in the ab sub-plane during the healthy operation
表2 开关表
Tab.2 Switch table
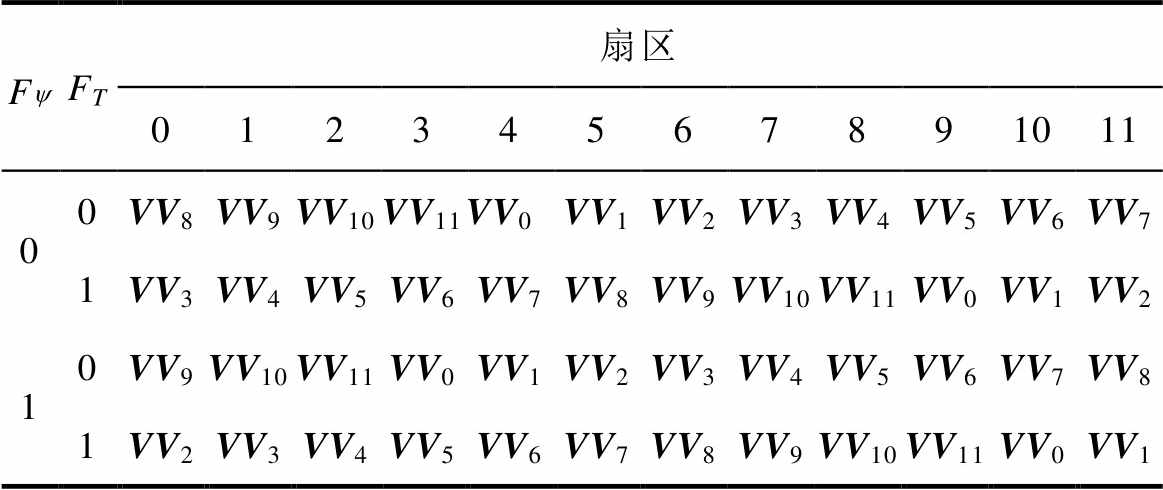
FyFT扇区 01234567891011 00VV8VV9VV10VV11VV0VV1VV2VV3VV4VV5VV6VV7 1VV3VV4VV5VV6VV7VV8VV9VV10VV11VV0VV1VV2 10VV9VV10VV11VV0VV1VV2VV3VV4VV5VV6VV7VV8 1VV2VV3VV4VV5VV6VV7VV8VV9VV10VV11VV0VV1
当双三相PMSM发生单相开路故障时,基本电压矢量由正常的64个变为32个,因此需要对缺相后的虚拟矢量进行重新修正。由图4所示,双三相电机单相开路故障后电压矢量幅值不再满足特定的比例关系,且空间分布也不再均匀,因此很难采用类似双三相电机正常运行时直接合成的方法进行虚拟矢量修正。本文提出一种间接的虚拟矢量修正方法。以F相发生开路故障为例,使修正后的虚拟矢量在z子平面的谐波电压仍为零,则参考电压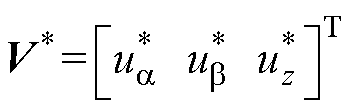 表示为[g cosq g sinq 0]T。其中,g 为ab 子平面参考电压的幅值,q表示ab 子平面参考电压矢量与a 轴的夹角。缺相故障后系统不再对称,无法注入零序电压,因此相电压与极电压相等,即相电压的幅值需要小于0.5Udc。通过对式(5)进行分析可以得出当ab 子平面线性调制比m=0.277 3(m=|vab|/Udc)时,剩余五相参考相电压的最大幅值正好为0.5Udc,即在保证z子平面谐波电压为零的前提下,ab 子平面在任意幅值和相位下都可以合成幅值为0.277 3Udc的电压矢量。图6为参考电压矢量
表示为[g cosq g sinq 0]T。其中,g 为ab 子平面参考电压的幅值,q表示ab 子平面参考电压矢量与a 轴的夹角。缺相故障后系统不再对称,无法注入零序电压,因此相电压与极电压相等,即相电压的幅值需要小于0.5Udc。通过对式(5)进行分析可以得出当ab 子平面线性调制比m=0.277 3(m=|vab|/Udc)时,剩余五相参考相电压的最大幅值正好为0.5Udc,即在保证z子平面谐波电压为零的前提下,ab 子平面在任意幅值和相位下都可以合成幅值为0.277 3Udc的电压矢量。图6为参考电压矢量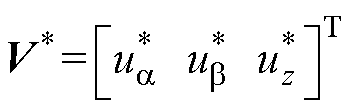 为[0.2773Udccosq 0.2773Udcsinq 0]T,即uz=0,ab 子平面线性调制比m=0.277 3时根据式(5)的逆变换得到的各相参考电压波形。
为[0.2773Udccosq 0.2773Udcsinq 0]T,即uz=0,ab 子平面线性调制比m=0.277 3时根据式(5)的逆变换得到的各相参考电压波形。
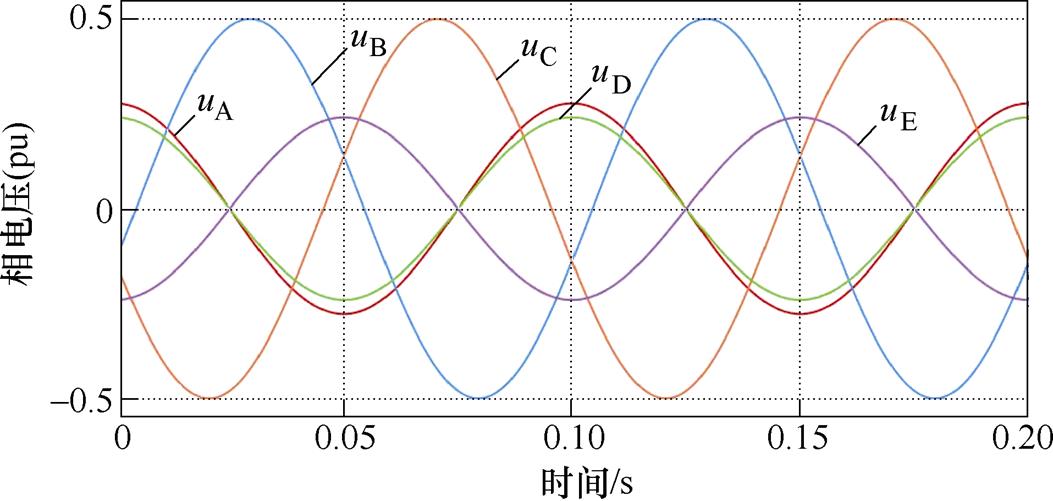
图6 ab 子平面线性调制比m=0.277 3时各相参考电压
Fig.6 Reference phase voltages when the linear modulation ratio m=0.277 3 in the absub-plane
为了便于控制,假定双三相PMSM单相故障时的虚拟矢量与正常运行时的方向一致,以虚拟矢量
为例,此时g =0.277 3Udc,q=p/12,即此时参考电压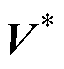 =[0.277 3Udccos(p/12) 0.277 3Udcsin(p/12) 0]T,根据式(5)的逆变换可以得到各相参考电压参考值uA、uB、uC、uD、uE分别为0.267 8Udc、-0.009 6Udc、-0.258 2Udc、0.232 0Udc、-0.232 0Udc。由于缺相故障后不再注入零序电压,相电压与极电压相等,各相极电压与相占空比之间存在关系
=[0.277 3Udccos(p/12) 0.277 3Udcsin(p/12) 0]T,根据式(5)的逆变换可以得到各相参考电压参考值uA、uB、uC、uD、uE分别为0.267 8Udc、-0.009 6Udc、-0.258 2Udc、0.232 0Udc、-0.232 0Udc。由于缺相故障后不再注入零序电压,相电压与极电压相等,各相极电压与相占空比之间存在关系
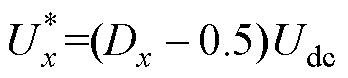 (9)
(9)
式中,x表示A、B、C、D、E;Dx为x相占空比。
利用式(9)可得到各相桥臂的占空比:DA、DB、DC、DD、DE分别为0.767 8、0.490 4、0.241 8、0.732 0、0.268 0。进而得到虚拟矢量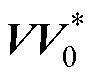 对应的PWM波形。以
对应的PWM波形。以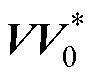 为例,g =0.2773Udc,q =p/12,其所得PWM波形如图7所示。
为例,g =0.2773Udc,q =p/12,其所得PWM波形如图7所示。
由图7可知:虚拟矢量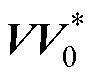 由4个基本电压矢量V16、V18、V26、V27合成,各个基本电压矢量所占比例分别为0.035 8、0.241 6、0.222 4、0.026 2。同理可以得到其余11个虚拟矢量所对应的基本电压矢量及其所占比例,进而得到双三相PMSM单相开路故障后十二个等幅虚拟矢量对应的基本矢量及其所占比例见表3。
由4个基本电压矢量V16、V18、V26、V27合成,各个基本电压矢量所占比例分别为0.035 8、0.241 6、0.222 4、0.026 2。同理可以得到其余11个虚拟矢量所对应的基本电压矢量及其所占比例,进而得到双三相PMSM单相开路故障后十二个等幅虚拟矢量对应的基本矢量及其所占比例见表3。
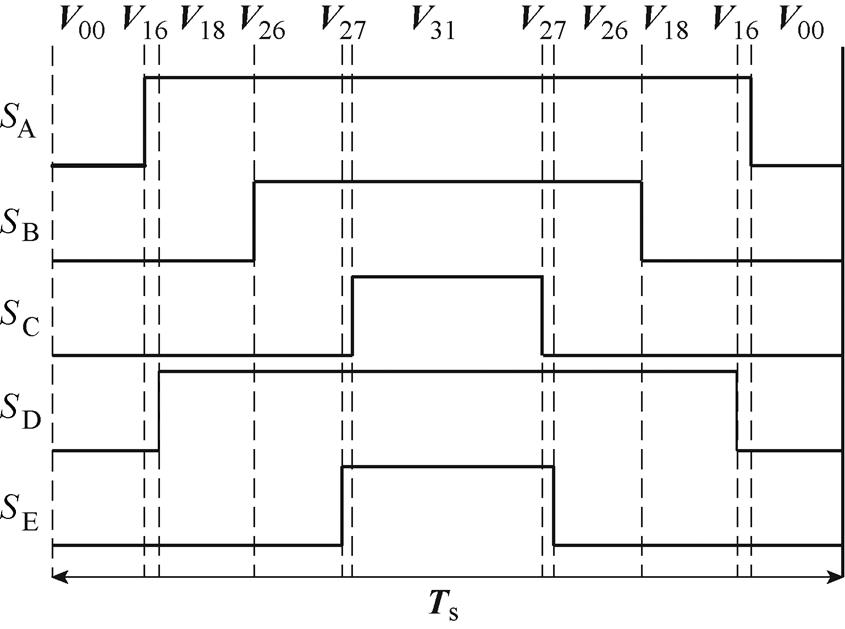
图7 单相开路故障时虚拟矢量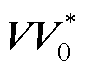 的PWM波形
的PWM波形
Fig.7 The PWM waveform of the virtual vector 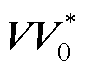 in one open-phase fault condition
in one open-phase fault condition
表3 单相开路故障后的等幅虚拟矢量所对应的基本电压矢量及其所占比例
Tab.3 The basic voltage vector and its proportion corresponding to the same amplitude virtual vector after one open-phase fault
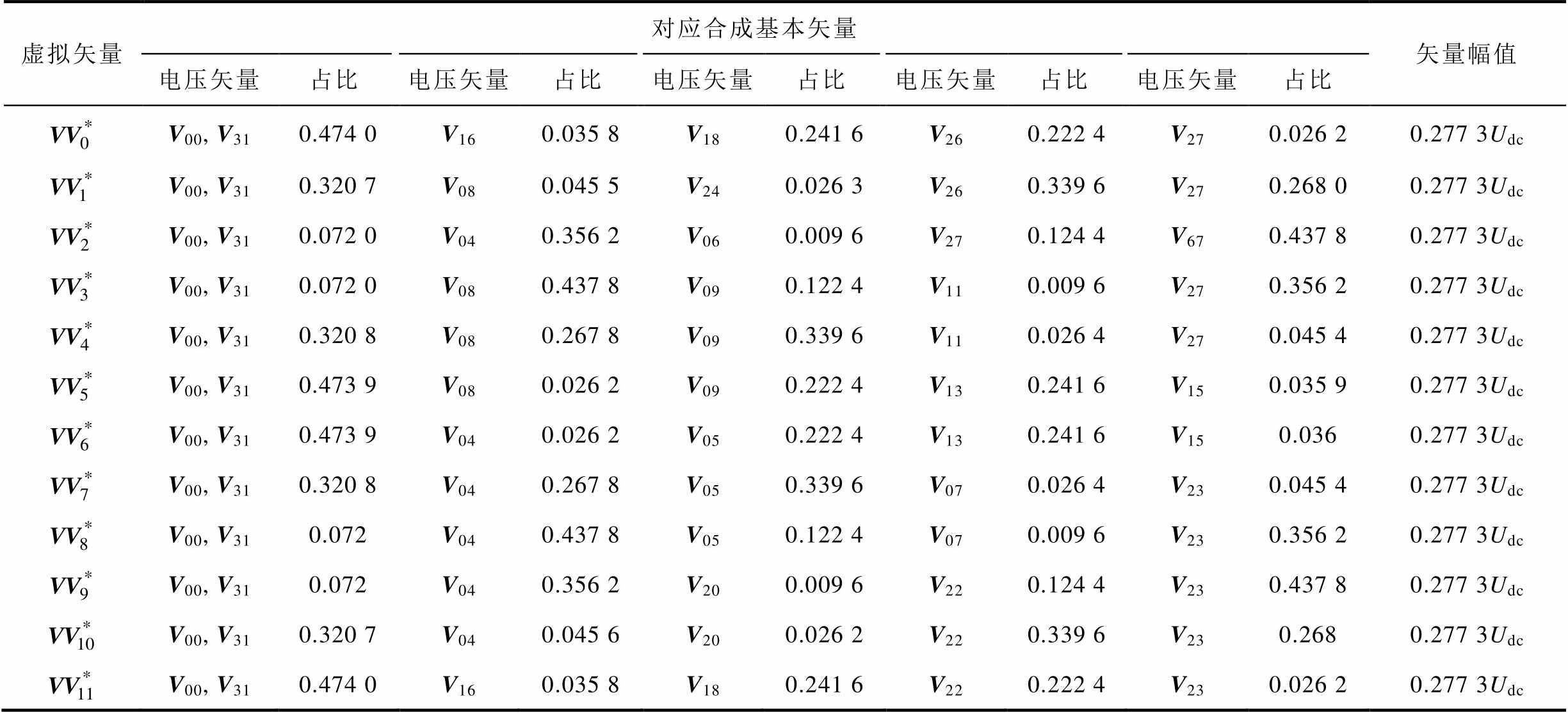
虚拟矢量对应合成基本矢量矢量幅值 电压矢量占比电压矢量占比电压矢量占比电压矢量占比电压矢量占比 V00, V310.474 0V160.035 8V180.241 6V260.222 4V270.026 20.277 3Udc V00, V310.320 7V080.045 5V240.026 3V260.339 6V270.268 00.277 3Udc V00, V310.072 0V040.356 2V060.009 6V270.124 4V670.437 80.277 3Udc V00, V310.072 0V080.437 8V090.122 4V110.009 6V270.356 20.277 3Udc V00, V310.320 8V080.267 8V090.339 6V110.026 4V270.045 40.277 3Udc V00, V310.473 9V080.026 2V090.222 4V130.241 6V150.035 90.277 3Udc V00, V310.473 9V040.026 2V050.222 4V130.241 6V150.0360.277 3Udc V00, V310.320 8V040.267 8V050.339 6V070.026 4V230.045 40.277 3Udc V00, V310.072V040.437 8V050.122 4V070.009 6V230.356 20.277 3Udc V00, V310.072V040.356 2V200.009 6V220.124 4V230.437 80.277 3Udc V00, V310.320 7V040.045 6V200.026 2V220.339 6V230.2680.277 3Udc V00, V310.474 0V160.035 8V180.241 6V220.222 4V230.026 20.277 3Udc
由表3可以看出,修正后的12个等幅虚拟矢量所对应的电压矢量中均含有一定比例的零矢量(V00和V31),零矢量的存在表明系统没有实现最大的直流母线电压利用率。因此通过减小零矢量的作用时间可进一步提高这12个虚拟矢量的幅值,当零矢量作用时间为零时对应该虚拟矢量的幅值达到最大为|Vmax|。
将表3中虚拟矢量的零矢量作用时间按相应比例分配给其余四个基本矢量,则有
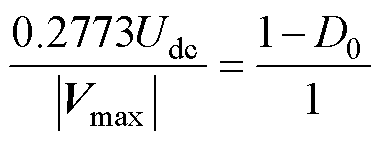 (10)
(10)
式中,|Vmax|为优化后不含零矢量的虚拟矢量幅值;D0为零矢量(V00, V31)的作用时间。
以优化前虚拟矢量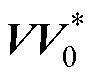 为例,D0=0.474,可得|Vmax|=0.527 2Udc,优化后的四个基本电压矢量V16、V18、V26、V27的作用时间分别为0.068 1、0.459 3、0.422 8、0.049 8。其余11个虚拟矢量按照同样的方法也可以得到最大幅值虚拟矢量。12个修正后的最大幅值虚拟矢量对应的基本矢量及其所占比例见表4。由表4可知,优化后的最大幅值虚拟矢量仅由四个非零基本电压矢量合成。以最大幅值虚拟矢量
为例,D0=0.474,可得|Vmax|=0.527 2Udc,优化后的四个基本电压矢量V16、V18、V26、V27的作用时间分别为0.068 1、0.459 3、0.422 8、0.049 8。其余11个虚拟矢量按照同样的方法也可以得到最大幅值虚拟矢量。12个修正后的最大幅值虚拟矢量对应的基本矢量及其所占比例见表4。由表4可知,优化后的最大幅值虚拟矢量仅由四个非零基本电压矢量合成。以最大幅值虚拟矢量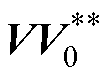 为例,其在ab 子平面和z子平面的矢量合成过程如图8所示。双三相PMSM系统F相开路故障后在ab 子平面的12个最大幅值虚拟矢量如图9 所示。
为例,其在ab 子平面和z子平面的矢量合成过程如图8所示。双三相PMSM系统F相开路故障后在ab 子平面的12个最大幅值虚拟矢量如图9 所示。
对比图5(正常运行)和图9(缺相故障运行)时的虚拟矢量可以看出12个虚拟矢量的方向完全一致,只是缺相故障运行时虚拟矢量的幅值有所减少,因此双三相电机缺相故障与正常直接转矩控制时其定子磁链分区和开关表的选取规则一致,不需要重新进行定子磁链分区和开关表设计,即本文构建的双三相电机缺相故障直接转矩控制运行策略与正常直接转矩控制运行时控制策略基本一致,其差别只是虚拟矢量的幅值有所减少,比较易于实现。双三相永磁同步电机缺相容错直接转矩控制算法框图如图10所示。开关表与正常DTC控制时开关表2一致,只是电压矢量选用单相开路故障后的虚拟矢量表3(等幅虚拟矢量)或表4(最大幅值虚拟矢量),其中等幅虚拟矢量,幅值相等但相对较小,适合低速轻载情况(相电流THD和转矩脉动较小);最大幅值虚拟矢量幅值不相等,但可以提高直流母线电压利用率,从而得到较大的速度和转矩输出,适合高速重载情况。定子磁链估算采用反电动势积分算法的电压模型,即式中,Rs为定子电阻;ysa、ysb 分别为定子磁链在a、b 轴上的分量;ya0、yb0分别为转子磁链在定子a、b 轴上的初始磁链;j 为定子磁链角。
表4 单相开路故障后的最大幅值虚拟矢量所对应的基本电压矢量及其占比
Tab.4 The basic voltage vector and its proportion corresponding to the maximum amplitude virtual vector after one open-phase fault
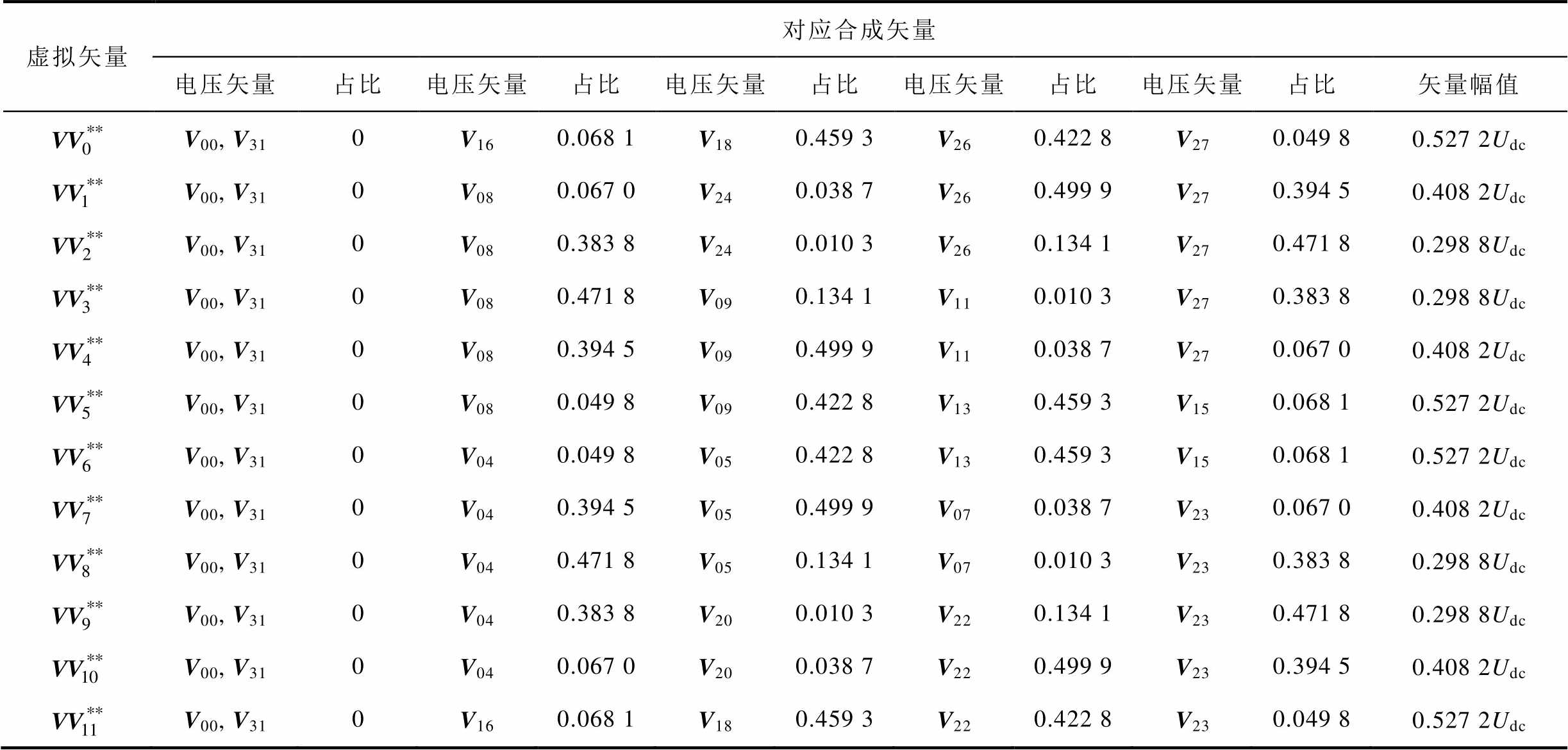
虚拟矢量对应合成矢量 电压矢量占比电压矢量占比电压矢量占比电压矢量占比电压矢量占比矢量幅值 V00, V310V160.068 1V180.459 3V260.422 8V270.049 80.527 2Udc V00, V310V080.067 0V240.038 7V260.499 9V270.394 50.408 2Udc V00, V310V080.383 8V240.010 3V260.134 1V270.471 80.298 8Udc V00, V310V080.471 8V090.134 1V110.010 3V270.383 80.298 8Udc V00, V310V080.394 5V090.499 9V110.038 7V270.067 00.408 2Udc V00, V310V080.049 8V090.422 8V130.459 3V150.068 10.527 2Udc V00, V310V040.049 8V050.422 8V130.459 3V150.068 10.527 2Udc V00, V310V040.394 5V050.499 9V070.038 7V230.067 00.408 2Udc V00, V310V040.471 8V050.134 1V070.010 3V230.383 80.298 8Udc V00, V310V040.383 8V200.010 3V220.134 1V230.471 80.298 8Udc V00, V310V040.067 0V200.038 7V220.499 9V230.394 50.408 2Udc V00, V310V160.068 1V180.459 3V220.422 8V230.049 80.527 2Udc
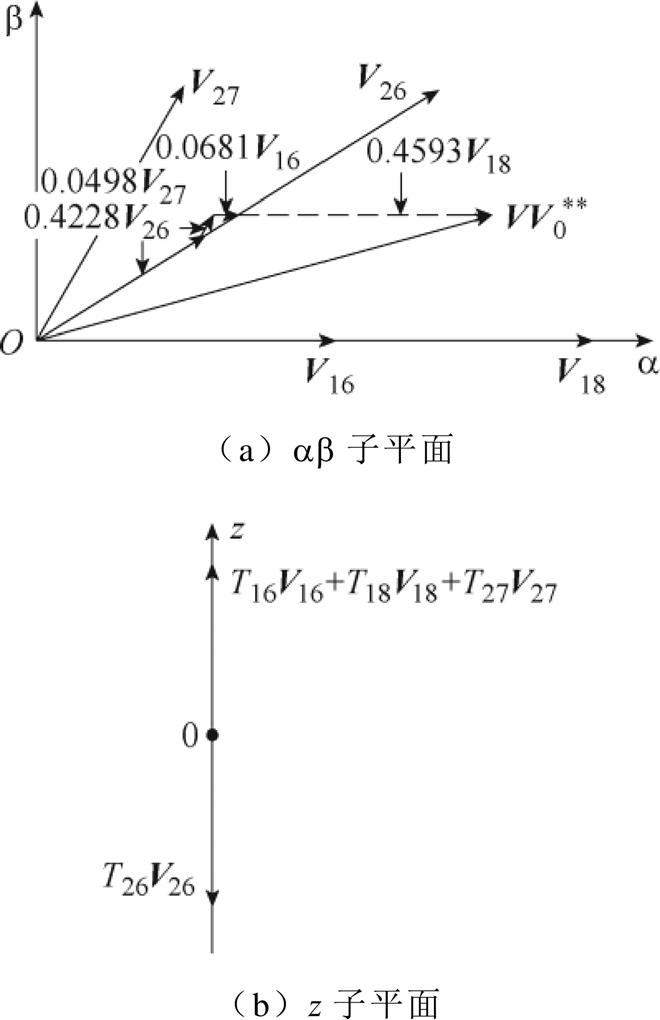
图8 虚拟矢量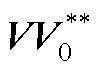 在ab 和z子平面的矢量合成图
在ab 和z子平面的矢量合成图
Fig.8 The vector composite diagram of the virtual vector 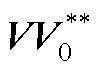 in the ab and z sub-planes
in the ab and z sub-planes
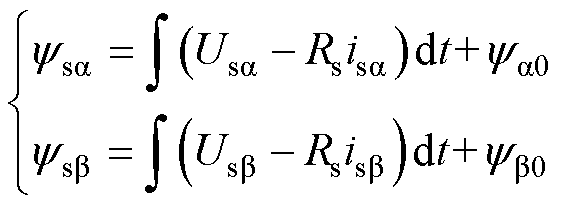 (11)
(11)
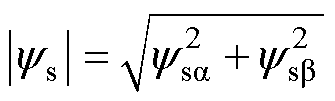 (12)
(12)
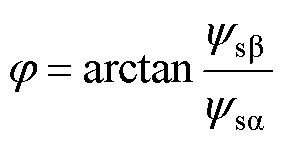 (13)
(13)
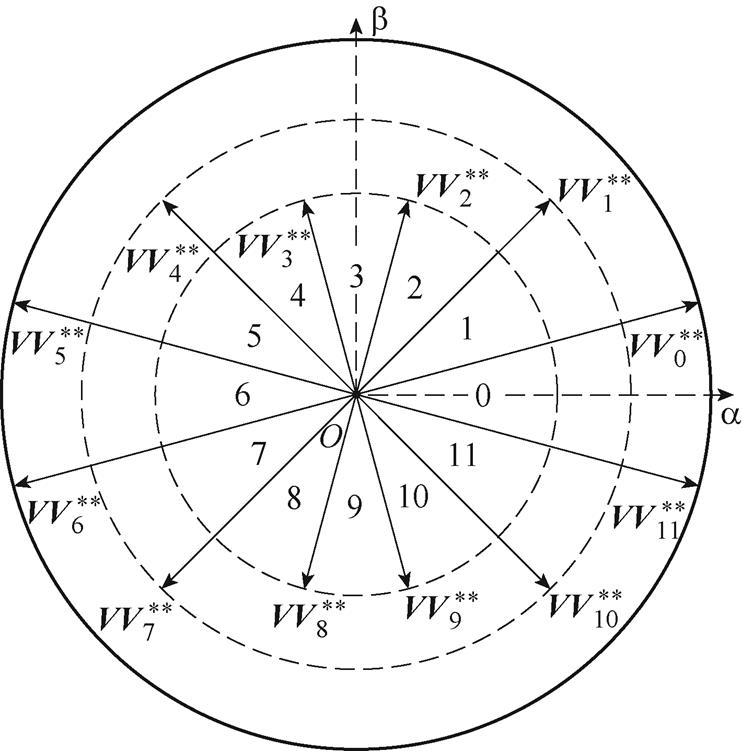
图9 单相开路故障后ab 子平面上的最大幅值虚拟矢量分布图
Fig.9 The maximum amplitude virtual vector distribution diagram in the ab sub-plane under the fault condition
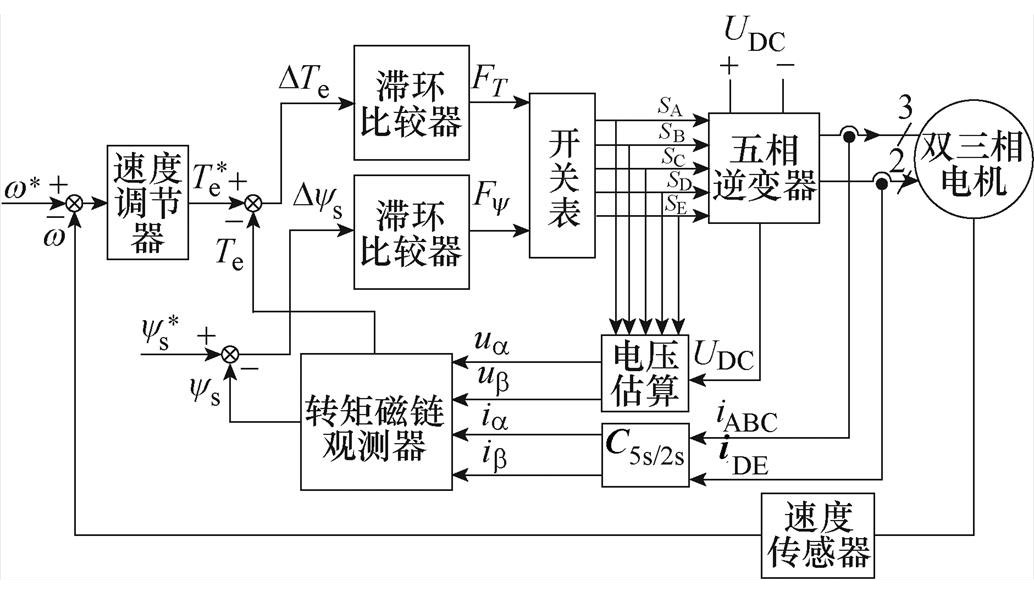
图10 基于虚拟矢量的容错型DTC算法框图
Fig.10 Block diagram of fault-tolerant DTC algorithm based on virtual vector
为验证本文提出控制策略的可行性,在一台表贴式双三相PMSM上进行实验验证。电机参数为:定子电阻0.5W,直交轴电感2.04mH,永磁体磁链0.12Wb,极对数为4。实验平台如图11所示。控制器采用Infineon公司的XE164高性能微控制器,逆变器采用英飞凌公司的FF300R12ME4功率模块。采用直流电机作为负载,控制频率为10kHz,死区时间为2ms,直流母线电压为200V。
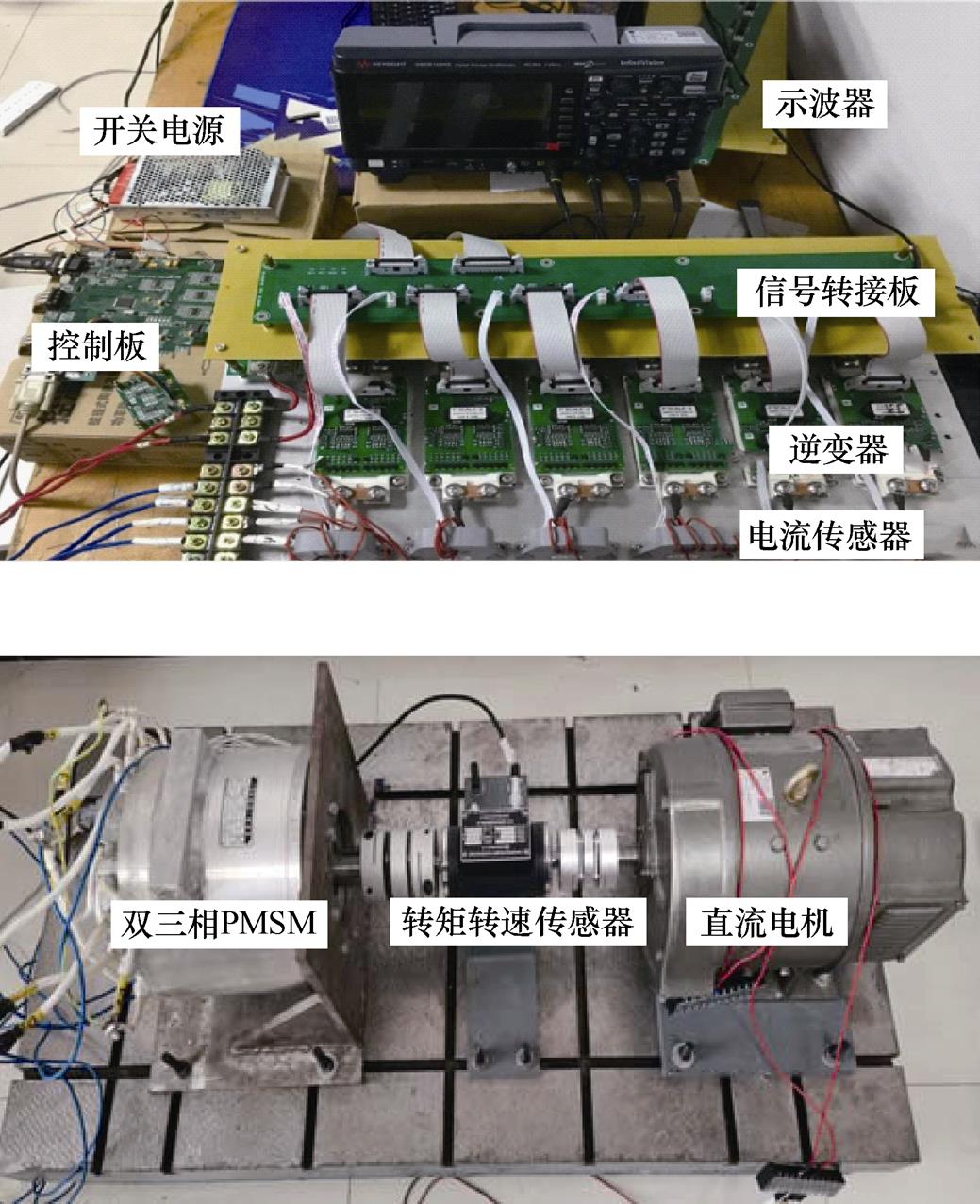
图11双三相PMSM驱动系统实验平台
Fig.11 Dual three-phase PMSM drive system prototype platform
图12为双三相电机正常运行时的相电流、转矩和转速波形。电机负载恒定为7N·m,转速恒定为200r/min。双三相电机正常运行时A相相电流的THD为5.6%,转矩脉动为1N∙m。
实验中使F相开路故障,电机负载恒定为7N·m,转速恒定为200r/min。图13为双三相电机正常运行、未使用虚拟矢量(缺一相)、等幅虚拟矢量(缺一相)和最大幅值虚拟矢量(缺一相)四种控制方式下谐波电流iz和转矩脉动的比较波形,不同控制方式下的A相相电流THD和转矩脉动见表5。未使用虚拟矢量(缺一相)方式为双三相电机缺一相故障后采用图4中的原始矢量(V18、V26、V27、V11、V09、V13、V05、V07、V23、V22)进行直接转矩控制,即未对电压矢量进行修正,由于原始矢量在z平面的电压幅值不为零,因此此时谐波电流较大,由图13b所示A相相电流的THD为141.8%,转矩脉动为1.7N∙m。图13c为双三相电机缺一相故障后采用表3中的等幅虚拟矢量进行直接转矩控制时的波形,A相相电流的THD为7.8%,转矩脉动为1.2N∙m。比双三相电机正常运行时略大,与图13b未使用虚拟矢量(缺一相)方式相比谐波电流得到了明显的抑制。图13d为双三相电机缺一相故障后采用表4中的最大幅值虚拟矢量进行直接转矩控制时的波形,A相相电流的THD为15.7%,转矩脉动为1.4N∙m,与图13c等幅虚拟矢量(缺一相)方式相比最大幅值虚拟矢量(缺一相)谐波电流和转矩脉动都有所增大,主要是由于最大幅值虚拟矢量幅值较大且不同方向上的幅值不相等,但最大幅值虚拟矢量可以提高直流母线电压利用率。因此在低速轻载工况场合下采用等幅虚拟矢量可以得到较小的相电流THD和转矩脉动,在高速重载工况场合下需要采用最大幅值虚拟矢量,从而得到较大的速度和转矩输出。
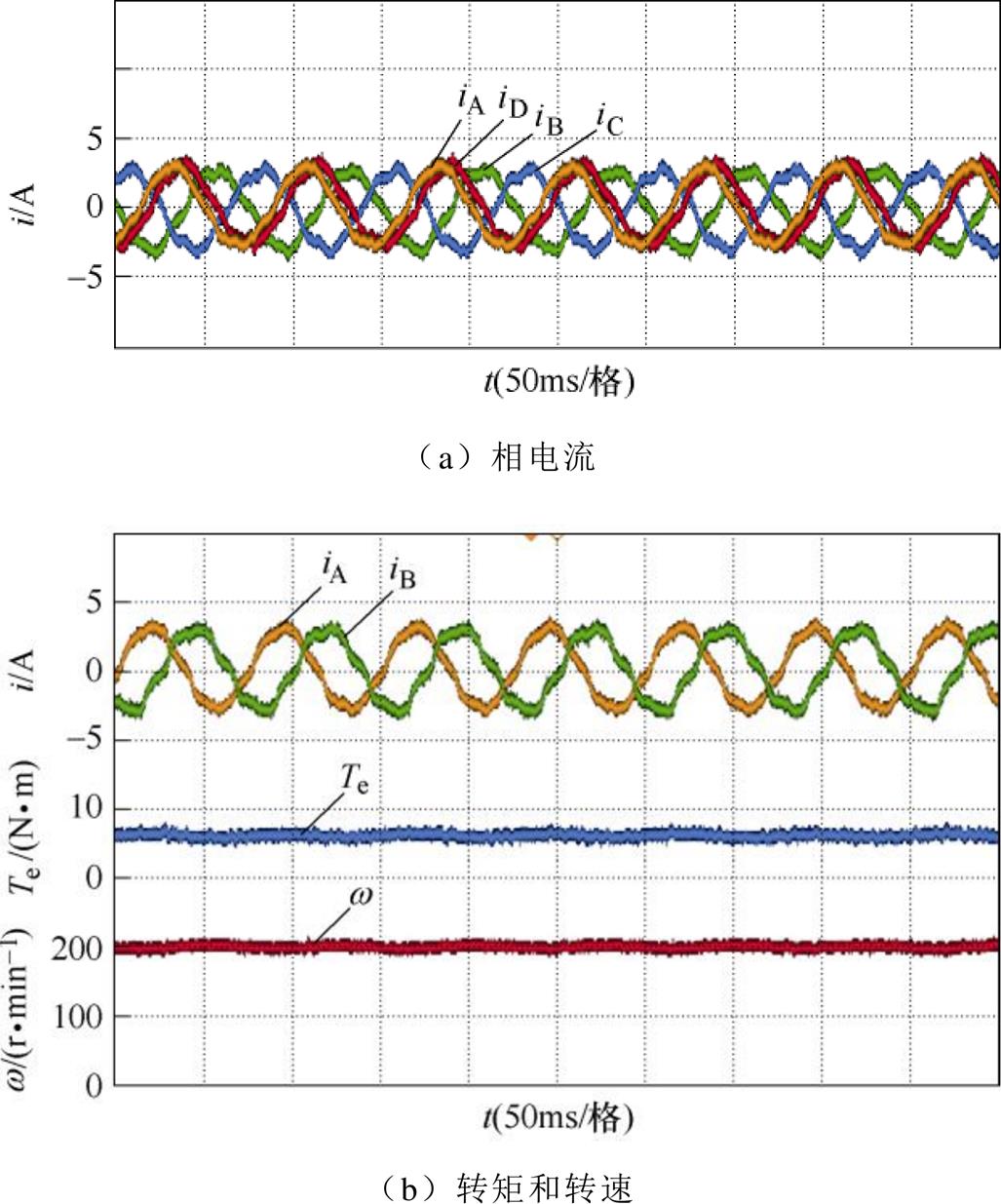
图12 正常运行时的实验结果
Fig.12 Experimental results of the normal mode
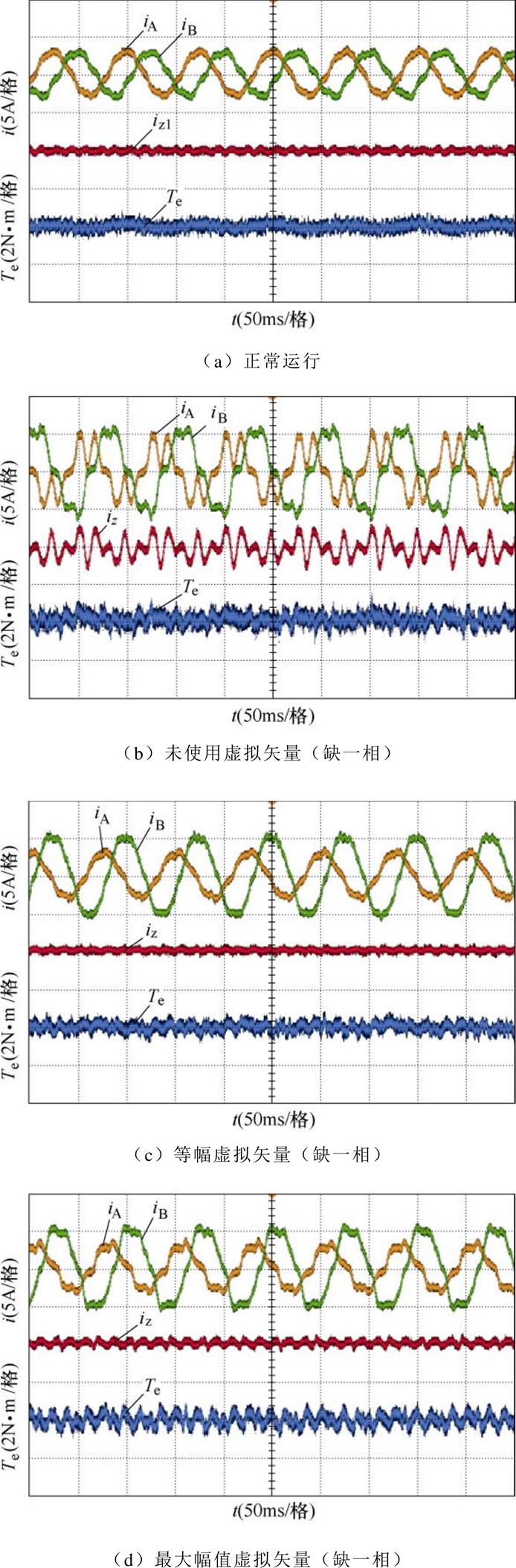
图13 不同控制方式下谐波电流和转矩脉动比较
Fig.13 Harmonic currents and torque pulsations in different control methods
表5 不同控制方式下的相电流THD和转矩脉动
Tab.5 Phase currents THD and torque pulsations in different control methods
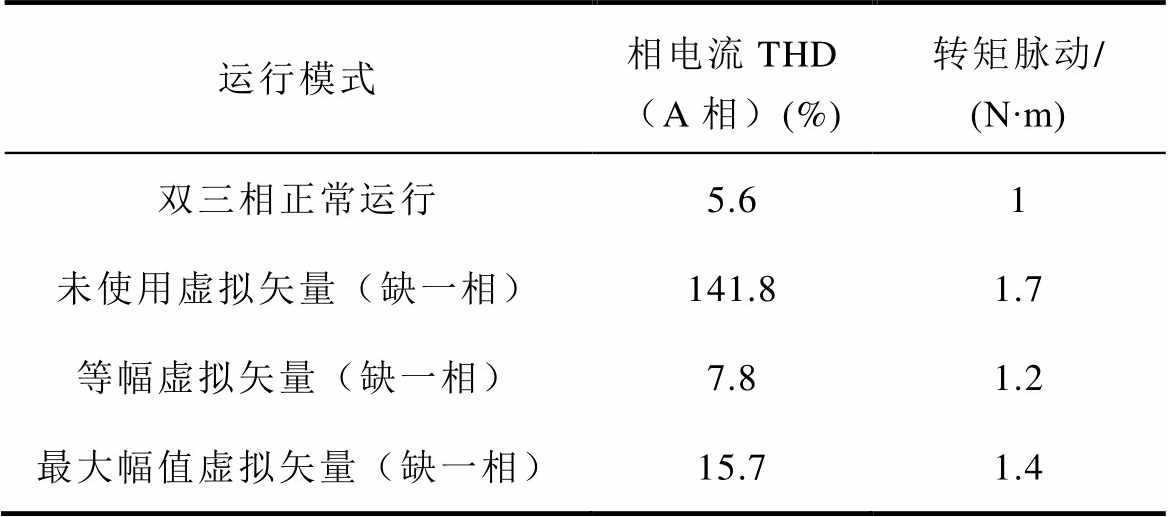
运行模式相电流THD(A相)(%)转矩脉动/ (N∙m) 双三相正常运行5.61 未使用虚拟矢量(缺一相)141.81.7 等幅虚拟矢量(缺一相)7.81.2 最大幅值虚拟矢量(缺一相)15.71.4
由于本文实验条件为低速轻载工况,因此以下实验均采用等幅虚拟矢量(缺一相)方式,图14为双三相电机采用等幅虚拟矢量缺相容错运行时的相电流、电磁转矩和转速波形,电机负载恒定为7N·m,转速恒定为200r/min。可以看出相电流正弦性良好,采用本文提出的缺一相容错直接转矩控制策略可以较好地抑制谐波电流和转矩脉动,且具有较好的稳态性能。图15为双三相电机从100r/min加速到300r/min时的相电流、电磁转矩和转速的实验波形。图16为负载转矩从3N∙m突加到7N∙m时对应的相电流、电磁转矩和转速实验波形,图17为负载转矩从7N∙m突减到3N∙m时对应的相电流、电磁转矩和转速实验波形,电机转速给定为200r/min。负载突变时,电机电磁转矩经过短暂调节就可以跟随给定,电机转速波动较小。可以看出系统的动态性能良好,且具有较好的抗干扰能力。
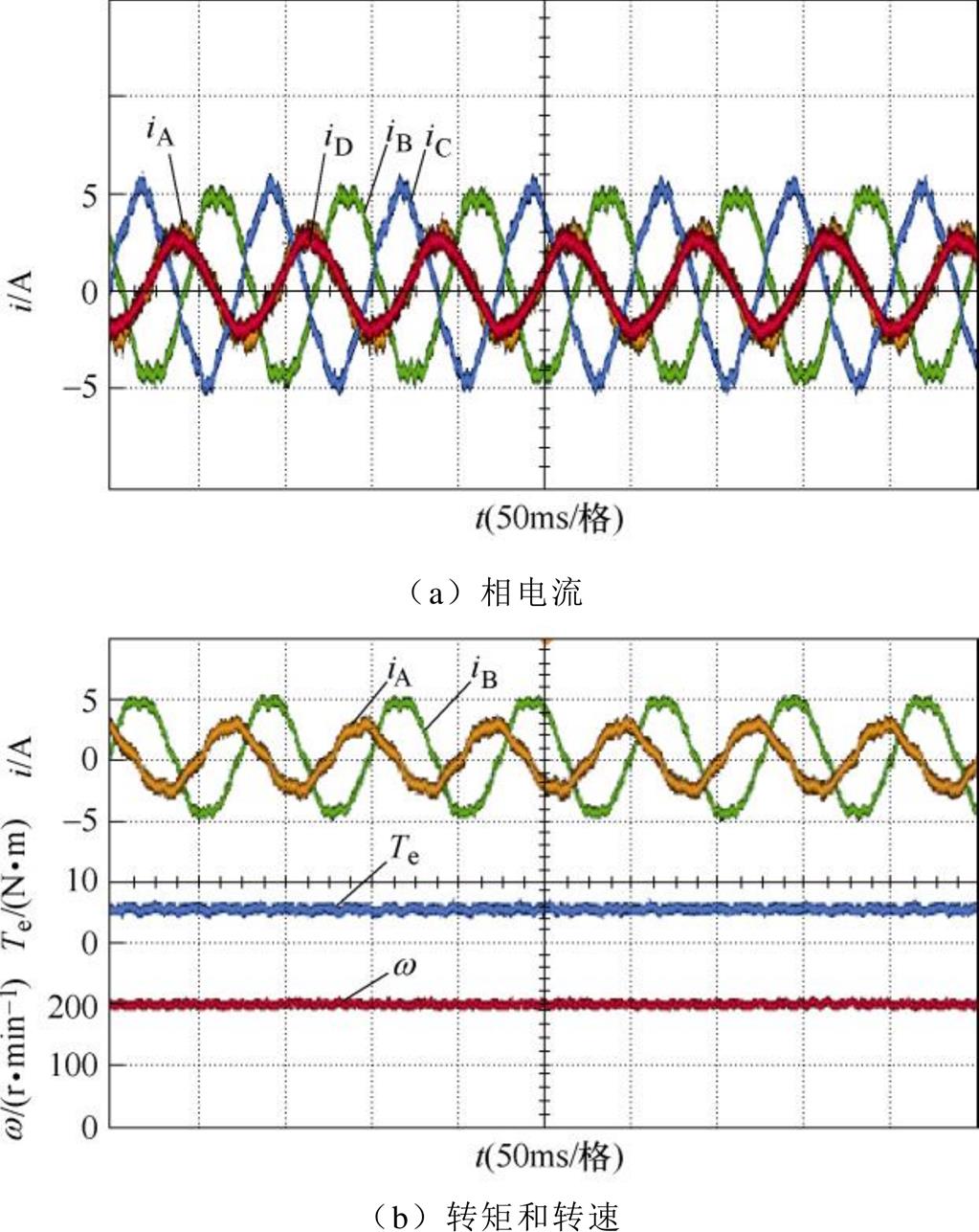
图14 等幅虚拟矢量容错运行
Fig.14 Experimental results of the same amplitude virtual vector method
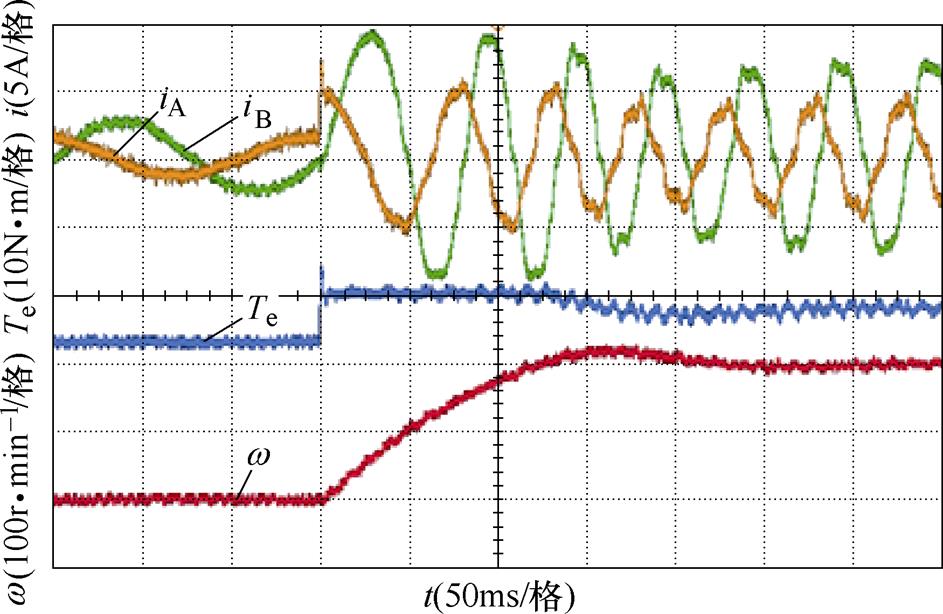
图15 电机加速时的实验结果
Fig.15 Experimental results of the motor acceleration process
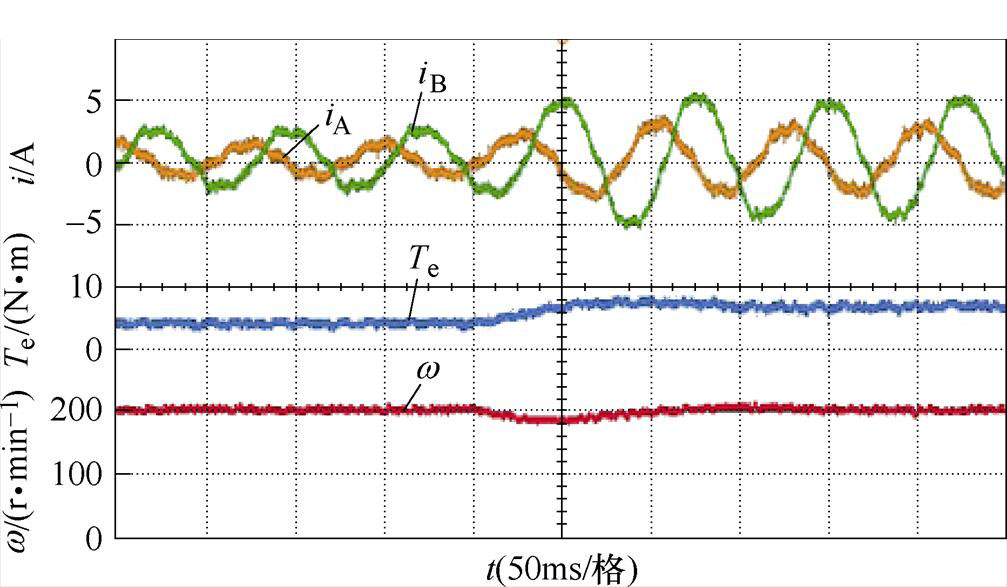
图16 负载突增时的实验结果
Fig.16 Experimental results when the load torque is suddenly increased
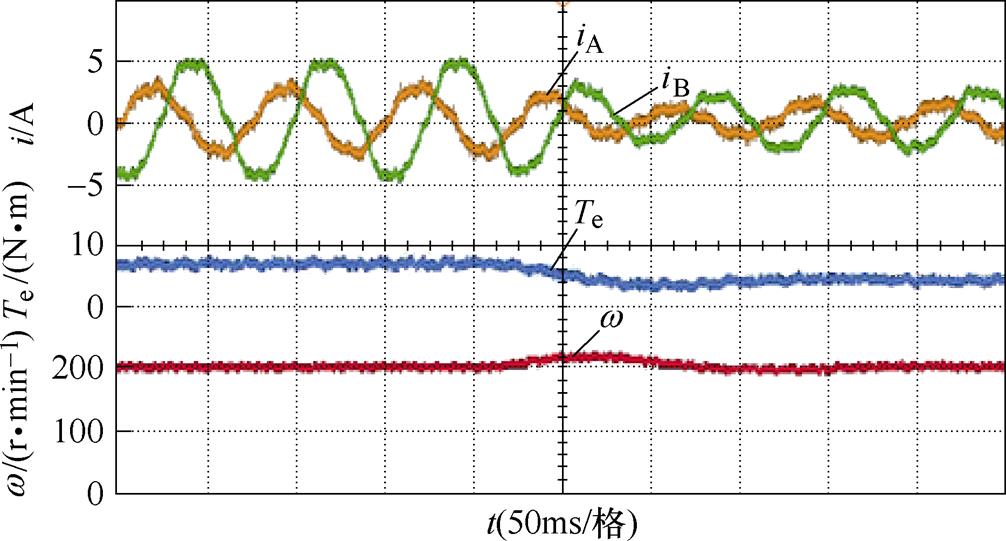
图17 负载突减时的实验结果
Fig.17 Experimental results when the load torque is suddenly reduced
图18为正反转实验波形,电机转速从200r/min突变到-200r/min。从实验结果可以看出,采用本文构建的容错直接转矩控制策略,双三相电机缺一相故障后可以稳定运行,且具有较好的动静态性能。图19为双三相电机从正常运行到故障运行的切换波形,可以看到当F相发生开路故障后,F相电流突变为零,剩余相电流发生较大畸变,同时具有较大的转矩脉动。图20为双三相电机从故障运行到容错直接转矩控制运行时的切换波形,可以看到采用本文构建的容错直接转矩控制策略可以有效抑制转矩脉动。
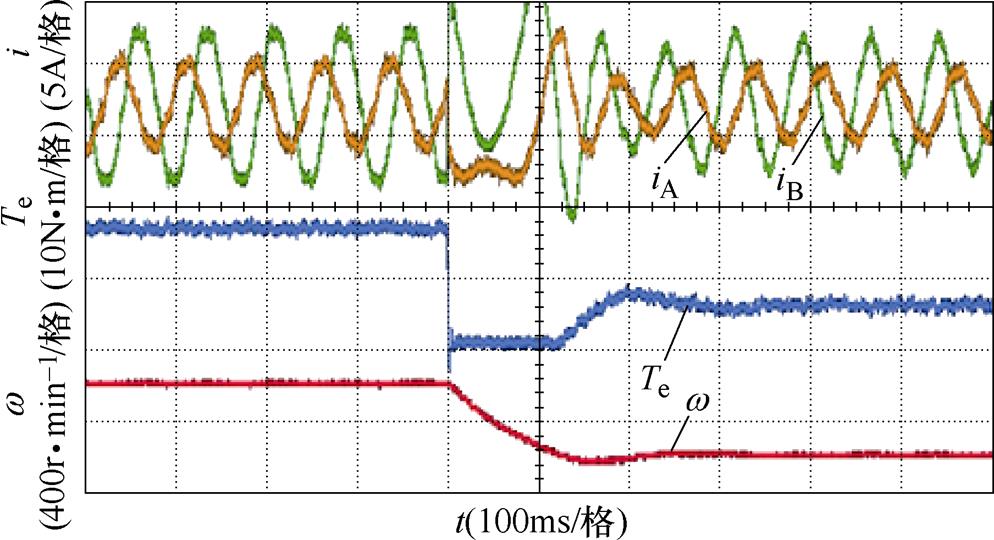
图18 电机正反转切换运行时的实验结果
Fig.18 Experimental results when the motor is switched from forward to reverse
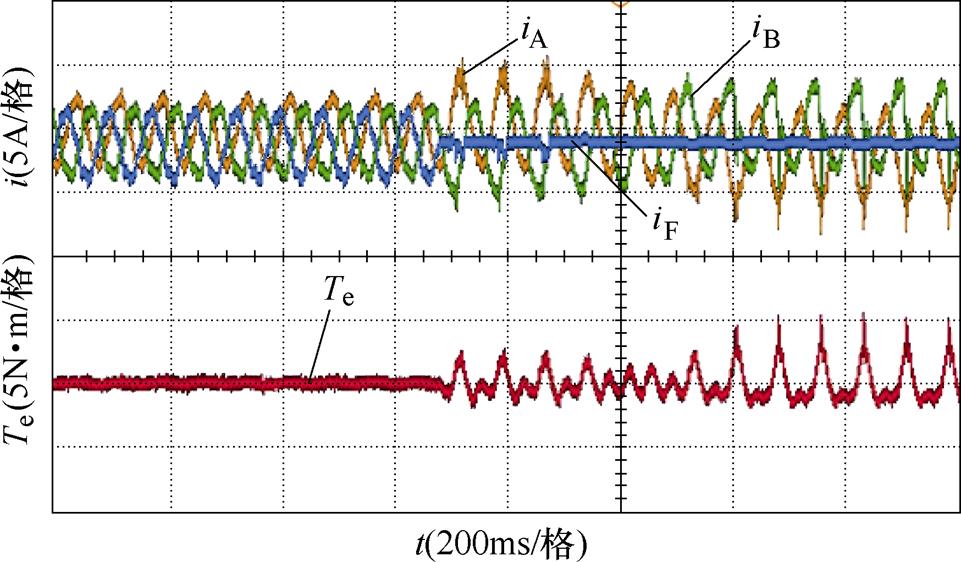
图19 正常运行到缺相故障运行时的切换波形
Fig.19 Switching waveforms from the normal operation to the open-phase fault operation
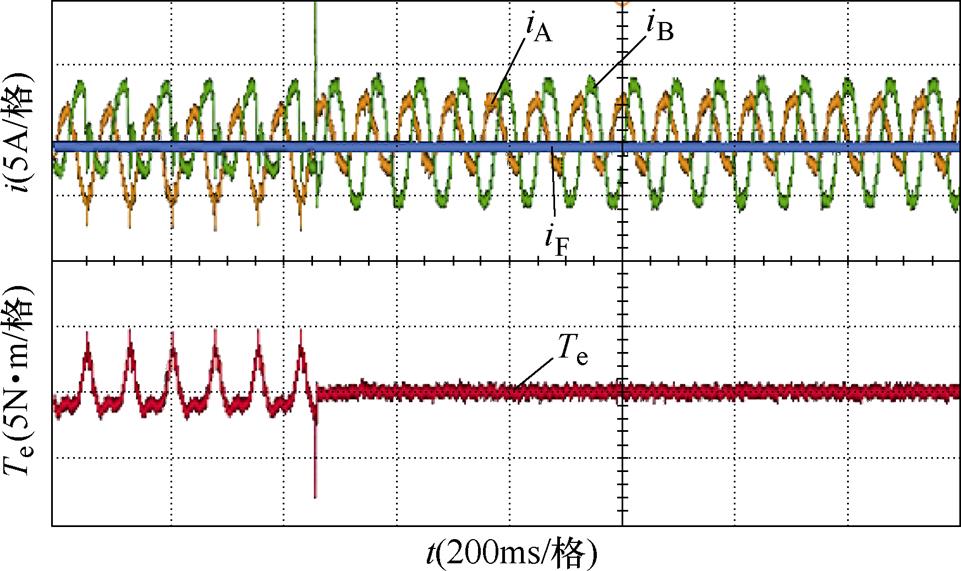
图20 缺相故障运行到容错运行时的切换波形
Fig.20 Switching waveforms from the open-phase fault operation to fault-tolerant operation
本文针对双三相永磁同步电机缺一相故障情况,主要提出了一种双三相电机缺相容错运行虚拟矢量的间接修正方法,并且构建了双三相电机缺一相容错直接转矩控制策略,得到以下结论:
1)双三相永磁同步电机缺一相直接转矩控制需要对虚拟矢量进行重新修正,采用修正后的虚拟矢量可以有效抑制故障运行时的转矩脉动,实现缺一相故障后的有效直接转矩控制。
2)本文提出的双三相PMSM缺一相故障运行虚拟矢量间接修正方法,可以得到等幅和最大幅值两种虚拟矢量,其中最大幅值虚拟矢量消除了零矢量,实现了最大直流母线电压利用。
3)本文构建的双三相电机缺一相直接转矩控制策略,其定子磁链分区和开关表的选取规则与双三相电机正常运行直接转矩控制一致,其差别只是12个虚拟矢量的幅值有所减少,因此比较易于实现。
4)本文提出的缺相容错运行虚拟电压矢量间接修正方法对于其他类型多相电机如五相电机,正常运行以及缺两相运行情况均适用,只需要分别采用对应运行情况下的静止变换阵即可。虚拟电压矢量也可以用于多相电机缺相容错运行时的SVPWM和预测电流控制中。
参考文献
[1] Levi E. Advances in converter control and innovative exploitation of additional degrees of freedom for multiphase machines[J]. IEEE Transactions on Indu- strial Electronics, 2016, 63(1): 433-448.
[2] Duran M J, Barrero F. Recent advances in the design, modeling, and control of multiphase machines—part II[J]. IEEE Transactions on Industrial Electronics, 2016, 63(1): 459-468.
[3] Duran M J, Barrero F. Recent advances in the design, modeling, and control of multiphase machines-part II[J]. IEEE Transactions on Industrial Electronics, 2016, 63(1): 459-468.
[4] 刘自程, 李永东, 郑泽东. 多相电机控制驱动技术研究综述[J]. 电工技术学报, 2017, 32(24): 17-29.
Liu Zicheng, Li Yongdong, Zheng Zedong. Control and drive techniques for multiphase machines: a review[J]. Transactions of China Electrotechnical Society, 2017, 32(24): 17-29.
[5] Suhel S M, Maurya R. A new switching sequences of SVPWM for six-phase induction motor with features of reduced switching losses[J]. CES Transactions on Electrical Machines and Systems, 2021, 5(2): 100- 107.
[6] 卜飞飞, 罗捷, 刘皓喆, 等. 双绕组感应发电机系统无差拍电流预测控制策略[J]. 电工技术学报, 2021, 36(24): 5213-5224.
Bu Feifei, Luo Jie, Liu Haozhe, et al. Deadbeat predictive current control strategy of dual winding induction generator system[J]. Transactions of China Electrotechnical Society, 2021, 36(24): 5213-5224.
[7] 蒋钱, 卢琴芬, 李焱鑫. 双三相永磁直线同步电机的推力波动及抑制[J]. 电工技术学报, 2021, 36(5): 883-892.
Jiang Qian, Lu Qinfen, Li Yanxin. Thrust ripple and depression method of dual three-phase permanent magnet linear synchronous motors[J]. Transactions of China Electrotechnical Society, 2021, 36(5): 883- 892.
[8] 韩一, 聂子玲, 许金, 等. 双三相非周期瞬态直线感应电机能量链切换控制策略[J]. 电工技术学报, 2021, 36(2): 258-267.
Han Yi, Nie Ziling, Xu Jin, et al. Energy chain switching control of a dual three-phase linear induction motor operating in non-periodic transient conditions[J]. Transactions of China Electrotechnical Society, 2021, 36(2): 258-267.
[9] 周长攀, 杨贵杰, 苏健勇, 等. 基于正常解耦变换的双三相永磁同步电机缺相容错控制策略[J]. 电工技术学报, 2017, 32(3): 86-96.
Zhou Changpan, Yang Guijie, Su Jianyong, et al. The control strategy for dual three-phase PMSM based on normal decoupling transformation under fault con- dition due to open phases[J]. Transactions of China Electrotechnical Society, 2017, 32(3): 86-96.
[10] 赵勇, 黄文新, 林晓刚, 等. 基于权重系数消除和有限控制集优化的双三相永磁容错电机快速预测直接转矩控制[J]. 电工技术学报, 2021, 36(1): 3-14.
Zhao Yong, Huang Wenxin, Lin Xiaogang, et al. Fast predictive direct torque control of dual three-phase permanent magnet fault tolerant machine based on weighting factor elimination and finite control set optimization[J]. Transactions of China Electrotechnical Society, 2021, 36(1): 3-14.
[11] 王学庆, 王政, 程明. T型三电平逆变器馈电双三相PMSM直接转矩控制[J]. 电工技术学报, 2017, 32(增刊1): 116-123.
Wang Xueqing, Wang Zheng, Cheng Ming. Direct torque control of T-type three-level inverter fed dual three-phase PMSM drives[J]. Transactions of China Electrotechnical Society, 2017, 32(S1): 116-123.
[12] Zheng Libo, Fletcher J E, Williams B W, et al. A novel direct torque control scheme for a sensorless five-phase induction motor drive[J]. IEEE Transa- ctions on Industrial Electronics, 2011, 58(2): 503- 513.
[13] Ren Yuan, Zhu Z Q. Reduction of both harmonic current and torque ripple for dual three-phase permanent- magnet synchronous machine using modified switching- table-based direct torque control[J]. IEEE Transa- ctions on Industrial Electronics, 2015, 62(11): 6671- 6683.
[14] 周长攀, 苏健勇, 杨贵杰. 双三相永磁同步电机直接转矩控制谐波电流抑制研究[J]. 电机与控制学报, 2015, 19(9): 46-53.
Zhou Changpan, Su Jianyong, Yang Guijie. Harmonic currents suppression in direct torque control of dual three-phase permanent magnet synchronous motor[J]. Electric Machines and Control, 2015, 19(9): 46-53.
[15] 黄林森, 赵文祥, 吉敬华, 等. 稳态性能改善的双三相永磁电机直接转矩控制[J]. 电工技术学报, 2022, 37(2): 355-367.
Huang Linsen, Zhao Wenxiang, Ji Jinghua, et al. Direct torque control for dual three-phase permanent- magnet machine with improved steady-state perfor- mance[J]. Transactions of China Electrotechnical Society, 2022, 37(2): 355-367.
[16] 耿乙文, 鲍宇, 王昊, 等. 六相感应电机直接转矩及容错控制[J]. 中国电机工程学报, 2016, 36(21): 5947-5956, 6039.
Geng Yiwen, Bao Yu, Wang Hao, et al. Direct torque and fault tolerant control for six-phase induction motor[J]. Proceedings of the CSEE, 2016, 36(21): 5947-5956, 6039.
[17] 陈浩, 和阳, 赵文祥, 等. 基于占空比调制的五相容错永磁游标电机直接转矩控制[J]. 电工技术学报, 2020, 35(5): 1055-1064.
Chen Hao, He Yang, Zhao Wenxiang, et al. Direct torque control of five-phase fault-tolerant permanent magnet vernier motor based on duty cycle modu- lation[J]. Transactions of China Electrotechnical Society, 2020, 35(5): 1055-1064.
[18] 武雪松, 宋文胜, 薛诚. 基于虚拟电压矢量集占空比优化的五相永磁同步电机直接转矩控制算法[J]. 中国电机工程学报, 2019, 39(3): 857-867, 964.
Wu Xuesong, Song Wensheng, Xue Cheng. Direct torque control schemes for five-phase permanent- magnet synchronous machines based on duty ratio optimization of virtual voltage vector sets[J]. Pro- ceedings of the CSEE, 2019, 39(3): 857-867, 964.
[19] Wang Xueqing, Wang Zheng, Xu Zhixian. A hybrid direct torque control scheme for dual three-phase PMSM drives with improved operation perfor- mance[J]. IEEE Transactions on Power Electronics, 2019, 34(2): 1622-1634.
[20] Bermudez M, Gonzalez-Prieto I, Barrero F, et al. Open-phase fault-tolerant direct torque control technique for five-phase induction motor drives[J]. IEEE Transactions on Industrial Electronics, 2017, 64(2): 902-911.
[21] Bermudez M, Gonzalez-Prieto I, Barrero F, et al. An experimental assessment of open-phase fault-tolerant virtual-vector-based direct torque control in five- phase induction motor drives[J]. IEEE Transactions on Power Electronics, 2018, 33(3): 2774-2784.
[22] 周扬忠, 程明, 陈小剑. 基于虚拟变量的六相永磁同步电机缺一相容错型直接转矩控制[J]. 中国电机工程学报, 2015, 35(19): 5050-5058.
Zhou Yangzhong, Cheng Ming, Chen Xiaojian. A tolerated fault direct torque control for six-phase permanent magnet synchronous motor with one opened phase based on fictitious variables[J]. Pro- ceedings of the CSEE, 2015, 35(19): 5050-5058.
[23] Chen Qian, Liu Guohai, Zhao Wenxiang, et al. Asymmetrical SVPWM fault-tolerant control of five-phase PM brushless motors[J]. IEEE Transa- ctions on Energy Conversion, 2017, 32(1): 12-22.
[24] Liu Guohai, Song Chengyan, Chen Qian. FCS-MPC- based fault-tolerant control of five-phase IPMSM for MTPA operation[J]. IEEE Transactions on Power Electronics, 2020, 35(3): 2882-2894.
[25] Tao Tao, Zhao Wenxiang, Du Yuxuan, et al. Simplified fault-tolerant model predictive control for a five-phase permanent-magnet motor with reduced computation burden[J]. IEEE Transactions on Power Electronics, 2020, 35(4): 3850-3858.
[26] 杨金波, 李铁才, 杨贵杰. 一相开路双三相永磁同步电机建模与控制[J]. 电工技术学报, 2011, 26(10): 167-173, 187.
Yang Jinbo, Li Tiecai, Yang Guijie. Modeling and control of dual three-phase PMSM with one open phase[J]. Transactions of China Electrotechnical Society, 2011, 26(10): 167-173, 187.
Abstract Multiphase machines have low voltage, high power, low torque pulsation, and good fault tolerance, which have good application prospects in high-power driving applications such as electric vehicles, marine electric propulsion, and wind power generation. The phase-shifted 30° dual three-phase motor (the asymmetric six-phase motor) has a greater application advantage due to the cancellation of the sixth torque harmonic among various multiphase machines. The high fault-tolerant capability is an important application feature of multiphase machines. Various open-circuit and short-circuit faults in the machine drive system can be converted into open-phase faults through fault isolation. Therefore, the research of fault-tolerant control strategies for multiphase machines is mainly focused on the open-phase fault. Currently, the common control strategies for fault-tolerant operation include field-oriented control (FOC), model predictive control, and direct torque control (DTC). Among them, DTC does not need complex coordinate transformation, which weakens the influence of motor parameter changes on the control effect. In addition, DTC also has the advantages of a simple structure and fast torque response. Thus, it is widely researched in the fault-tolerant control of multi-phase motors.
Firstly, the basic voltage vectors in the direct torque control for dual three-phase permanent magnet synchronous motor (PMSM) drives must be modified. Using the modified virtual vectors for DTC, the harmonic currents in the z1-z2 sub-plane can be suppressed significantly. When an open-phase fault occurs, the voltage vectors need to be modified again. The voltage vector distribution in the open-phase fault operation is introduced. An indirect correction method of virtual voltage vectors is proposed under the single-phase open-circuit fault, and the amplitudes of the virtual voltage vectors are maximized. Moreover, the single-phase open-circuit fault-tolerant direct torque control strategy for a dual three-phase motor based on virtual voltage vectors is constructed. Its stator flux chain partition and switching table selection rules are the same as the dual three-phase motor under healthy operation. The only difference is that the amplitudes of twelve virtual vectors are reduced in the open-phase fault operation.
Experimental verification was carried out on a surface-mounted dual three-phase PMSM. The parameters of the dual three-phase PMSM are listed as follows: Pn=4, Rs=0.5W, LD=2.04mH, LQ=2.04mH, and yfd=0.12Wb. The DC bus voltage is 200V. The switching frequency is 10kHz with a dead time of 2ms. Harmonic currents and torque pulsations in different control methods are compared and analyzed. The THD of phase current A is 5.6%, and the torque pulsation is 1N·m in the healthy DTC operation of dual three-phase PMSM. The THD of phase current A is 141.8%, and the torque pulsation is 1.7N·m under the single-phase open-circuit fault of dual three-phase PMSM when the original vectors are used. The THD of phase current A is 7.8%, and the torque pulsation is 1.2N·m in the open-phase fault DTC operation when the same amplitude virtual vectors are used. The THD of phase current A is 15.7%, and the torque pulsation is 1.4N·m in the open-phase fault DTC operation when the maximum amplitude virtual vectors are used. The amplitudes of the maximum amplitude virtual vectors are not equal, but the DC bus voltage utilization rate is improved. Compared with the same amplitude virtual vectors, the THD of phase currents and the torque pulsation are larger when the maximum amplitude virtual vectors are used. The same amplitude virtual vectors can be used under low speed and light load conditions for low harmonic currents and torque pulsations. The maximum amplitude virtual vectors can be used under high speed and heavy load conditions for larger speed and torque output.
The following conclusions can be drawn from the theoretical analysis and experimental results: ① An indirect correction method of virtual voltage vectors is proposed under the single-phase open-circuit fault, and the amplitudes of the virtual voltage vectors are maximized. ②The proposed control strategy ensures that the sector distribution and the selection rule of the look-up tables after the single-phase open-circuit fault are consistent with those in the healthy operation, simplifying the control process. ③ The virtual vectors indirect correction method proposed in this paper can also be extended to other multiphase motors, such as five-phase motors. And be applied to two-phase faults of motors.
Keywords:Dual three-phase permanent magnet synchronous motor, direct torque control, one phase open fault, virtual vector correction
中图分类号:TM301.2
DOI: 10.19595/j.cnki.1000-6753.tces.211702
国家自然科学基金(51707157)、中国博士后科学基金(2017M623210)和陕西省自然科学基础研究计划(2023-JC-YB-377)资助项目。
收稿日期 2021-10-28
改稿日期 2022-04-13
E-mail: zhoucp@xaut.edu.cn(通信作者)
刘海峰 男,1994年生,硕士研究生,研究方向为多相永磁同步电机驱动与控制。
E-mail: 1049734472@qq.com
(编辑 郭丽军)